
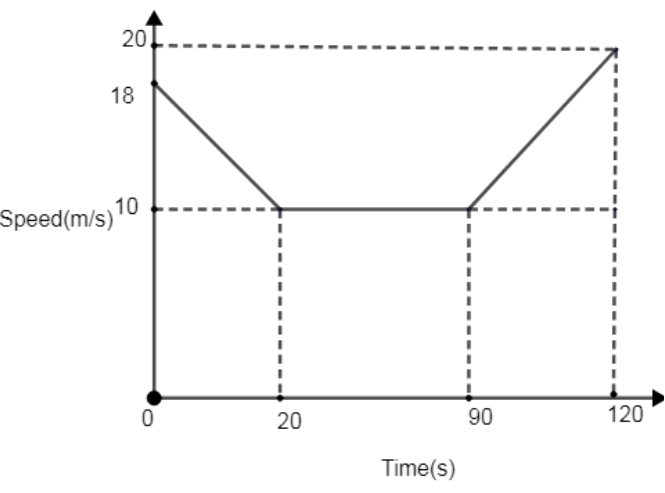
The diagram shows the speed-time graph for 120 seconds of a car journey.
(a) Calculate the deceleration of the car during the first 20 seconds.
(b) Calculate the total distance traveled by car during the 120 seconds.
(c) Calculate the average speed for this 120-second journey.

Answer
488.1k+ views
Hint: Divide the above journey of the car into 3 parts. The first part from 0 – 20 seconds, the second part from 20 – 90 seconds, and the third part from 90 – 120 seconds. For part (a), observe the graph from 0 – 20 seconds and apply the formula: -
Complete step-by-step solution
(a) We have to calculate the deceleration of the car during the first 20 seconds.
The term ‘deceleration’ means negative acceleration.
Applying the formula: -
Since, ‘a’ is negative, therefore deceleration =
(b) Here, we have to find the total distance traveled by car during the 120 seconds. Therefore, Total distance traveled = Area under the speed-time graph.
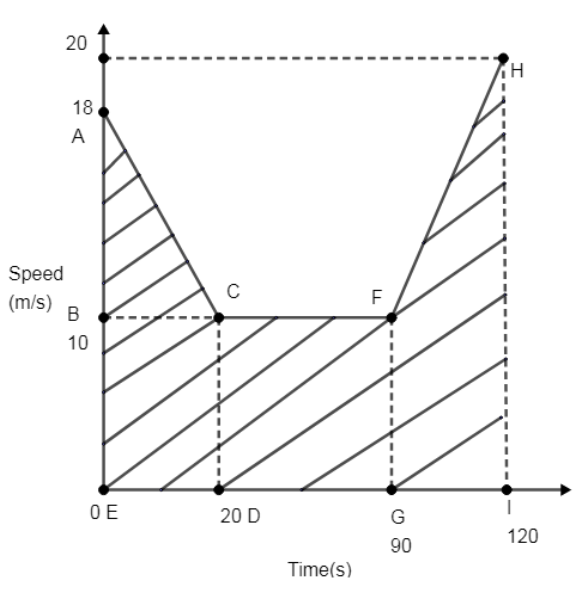
= Area of trapezium ABEDC + Area of rectangle CDGF +Area of trapezium FGIH
Applying the formula of area of trapezium =
We have, total distance travelled
= 1430m
Therefore, total distance travelled = 1430m.
(c) Here, we have to find the average speed during the 120 seconds journey.
Applying the formula for average speed =
We get, Average speed =
Hence, average speed during the 120s journey is nearly
Note: One may note that we have divided the journey into 3 parts because then it will be easy for us to calculate the area of the speed-time graph which is the total distance traveled. We can also calculate the area in different ways like by forming two triangles and a rectangle. The other method can include the method of integration which is used for complex curves, so we do not need such an integration method here.
Complete step-by-step solution
(a) We have to calculate the deceleration of the car during the first 20 seconds.
The term ‘deceleration’ means negative acceleration.
Applying the formula: -
Since, ‘a’ is negative, therefore deceleration =
(b) Here, we have to find the total distance traveled by car during the 120 seconds. Therefore, Total distance traveled = Area under the speed-time graph.

= Area of trapezium ABEDC + Area of rectangle CDGF +Area of trapezium FGIH
Applying the formula of area of trapezium =
We have, total distance travelled
= 1430m
Therefore, total distance travelled = 1430m.
(c) Here, we have to find the average speed during the 120 seconds journey.
Applying the formula for average speed =
We get, Average speed =
Hence, average speed during the 120s journey is nearly
Note: One may note that we have divided the journey into 3 parts because then it will be easy for us to calculate the area of the speed-time graph which is the total distance traveled. We can also calculate the area in different ways like by forming two triangles and a rectangle. The other method can include the method of integration which is used for complex curves, so we do not need such an integration method here.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE





