
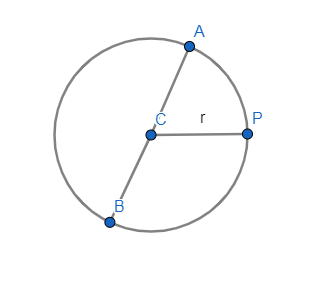
The diameter of a circle is a line which joins two points on the circle and also passes through the centre of the circle. (In the adjoining figure, AB is the diameter of the circle; C is its centre). Express the diameter of the circle (d) in terms of its radius (r).

Answer
519k+ views
1 likes
Hint: As the diameter is passing through centre of the circle and radius of the circle is a line joining from centre to a point on the circle and it is given that diameter is starting from a point on a circle and end on the other point on the circle passing through the centre so diameter looks as we have combined the two radii together side by side.
Complete step-by-step answer:
In the given figure, AB is the diameter and CP is the radius.
Diameter is a line segment starting from some point on the circle and ending at the other point on the circle passing through the centre of the circle. In the given question, AB is a diameter starting from point A and ending at point B passing through centre C.
Radius is a line segment starting from the centre of the circle and ending at some point on the circle. The diameter AB seems as CA is the radius of the circle and we have extended this radius from point C to B which is diametrically opposite to A then the line segment AB is the addition of two radii CA and CB.
AB = CA + CB
And it is given that the line segment AB is the diameter of the circle (d) and the value of radii CA and CB is (r) so substituting these values in the above relation we get:
d = r + r
Hence, the relation between d and r is d = 2r.
Note: Some properties of the diameter of the circle:
Diameter is the longest chord of the circle passing through the centre.
Angle subtended by the diameter of the circle at any point on the circle is 90°.
Complete step-by-step answer:
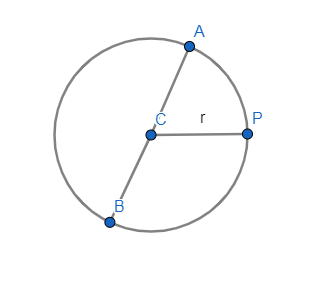
In the given figure, AB is the diameter and CP is the radius.

Diameter is a line segment starting from some point on the circle and ending at the other point on the circle passing through the centre of the circle. In the given question, AB is a diameter starting from point A and ending at point B passing through centre C.
Radius is a line segment starting from the centre of the circle and ending at some point on the circle. The diameter AB seems as CA is the radius of the circle and we have extended this radius from point C to B which is diametrically opposite to A then the line segment AB is the addition of two radii CA and CB.
AB = CA + CB
And it is given that the line segment AB is the diameter of the circle (d) and the value of radii CA and CB is (r) so substituting these values in the above relation we get:
d = r + r
Hence, the relation between d and r is d = 2r.
Note: Some properties of the diameter of the circle:
Diameter is the longest chord of the circle passing through the centre.
Angle subtended by the diameter of the circle at any point on the circle is 90°.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




