
The diameter of a road roller of length 120cm is 84cm. If it takes 500 complete revolutions to have a playground, then find the cost of levelling it at the cost of 75 paise per square meter. (Take
Answer
495.3k+ views
Hint: To solve the question given above, we will first find out the area of the playground to be levelled. For this we will calculate the area of the roller and multiply it with 500 to obtain the levelled area. Then, to find the cost of levelling, we will multiply the levelled area by 75 paise (Rs
Complete step by step answer:
It is given in the question that the roller is revolved 500 times to level the entire playground. So the total levelled area of the playground will be equal to 500 times the area of the roller. The shape of the roller is a cylinder. So, we have to basically find the curved area of the cylinder which has diameter =84cm and length 120cm. Thus, the radius of cylinder will be:
Let us draw the cylinder to get a better view.
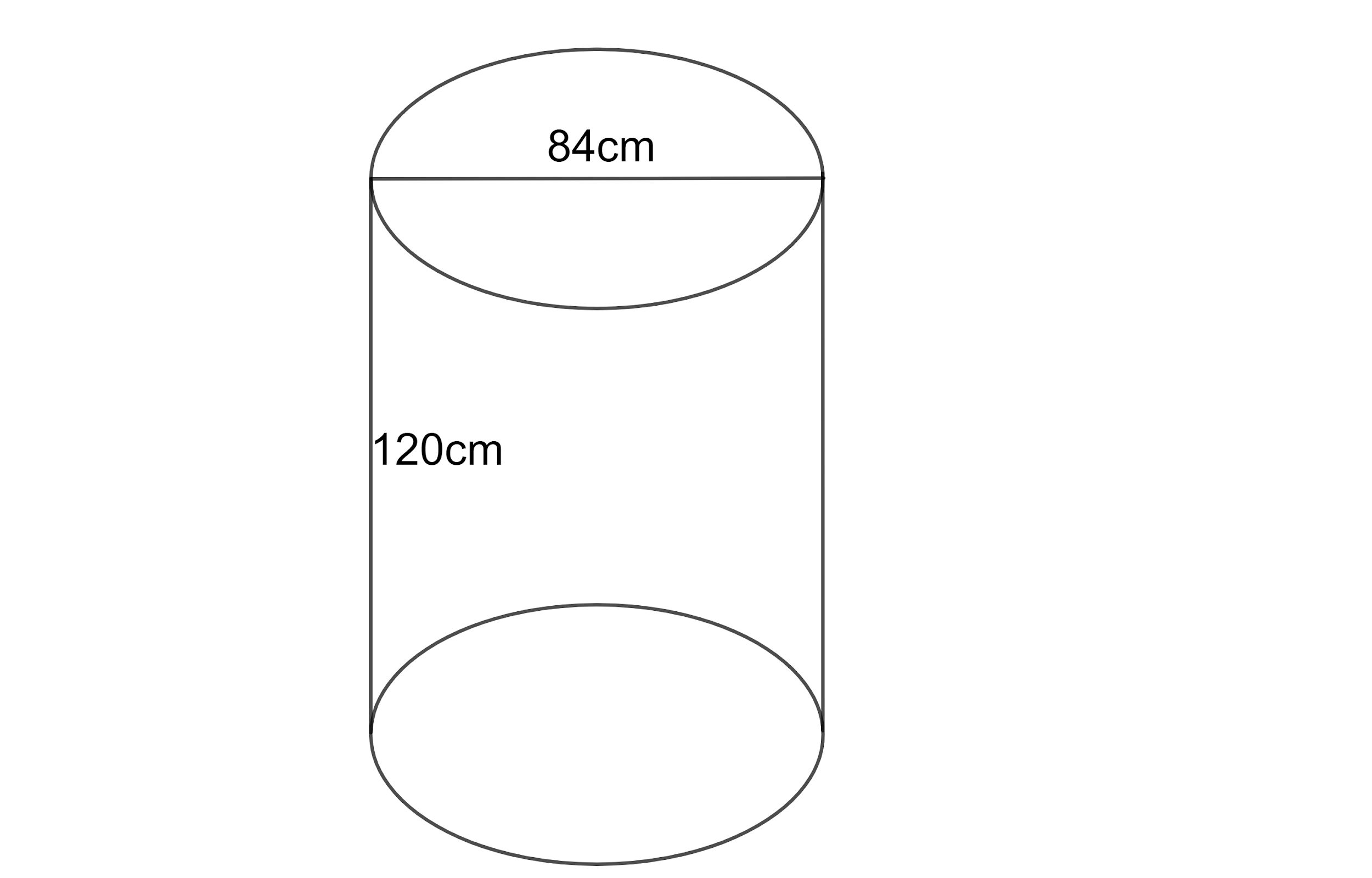
The curved surface of the cylinder with the radius and length given is calculated by the formula shown:
Curved surface area
Where ‘r’ is the radius of base and ‘l’ is the length of the cylinder.
Thus, the curved surface area of cylinder in our case will be:
Curved surface area
Now the area of the playground will be equal to 500 times the curved surface area of the cylinder.
So we have the following equation:
Area of playground
Now, we have to convert this area to
Thus, the area of the playground
Area of the playground
Now it is given that cost of levelling is 75 paise per square meter. Thus, the cost of 1 meter square is 75 paise.
Cost of
Cost of
Cost of
Now, we know that, 1 Rupee = 100 paise. Thus, we have:
Cost of
Cost of
Hence, the total cost of levelling is Rs 1188.
Note: We have assumed that the roller is cylindrical in shape i.e. the radius of the roller is constant everywhere and thus we have applied the area of the cylinder. If the radius is not constant, then the roller would be friction. Also, we have assumed that the cylinder is the right cylinder i.e. the axis of the cylinder is perpendicular to circular base.
Complete step by step answer:
It is given in the question that the roller is revolved 500 times to level the entire playground. So the total levelled area of the playground will be equal to 500 times the area of the roller. The shape of the roller is a cylinder. So, we have to basically find the curved area of the cylinder which has diameter =84cm and length 120cm. Thus, the radius of cylinder will be:
Let us draw the cylinder to get a better view.

The curved surface of the cylinder with the radius and length given is calculated by the formula shown:
Curved surface area
Where ‘r’ is the radius of base and ‘l’ is the length of the cylinder.
Thus, the curved surface area of cylinder in our case will be:
Curved surface area
Now the area of the playground will be equal to 500 times the curved surface area of the cylinder.
So we have the following equation:
Area of playground
Now, we have to convert this area to
Thus, the area of the playground
Area of the playground
Now it is given that cost of levelling is 75 paise per square meter. Thus, the cost of 1 meter square is 75 paise.
Cost of
Cost of
Cost of
Now, we know that, 1 Rupee = 100 paise. Thus, we have:
Cost of
Cost of
Hence, the total cost of levelling is Rs 1188.
Note: We have assumed that the roller is cylindrical in shape i.e. the radius of the roller is constant everywhere and thus we have applied the area of the cylinder. If the radius is not constant, then the roller would be friction. Also, we have assumed that the cylinder is the right cylinder i.e. the axis of the cylinder is perpendicular to circular base.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




