Question

The digit the units place in the cube root of 21952 is:
(a) 8
(b) 6
(c) 4
(d) 2

Answer 1

Hint: To solve the question given above, we will first find out the cube root of 21952 with the help of the prime factorization method. In the prime factorization method, we will write 21952 as the product of prime numbers. Then we will form a group of three of the same prime numbers and we will select one number from each group. The cube root of the number will be equal to the product of these numbers taken from each group. Then we will get the digit in the units place of that number as our final answer.

Complete step by step solution:
To find the unit’s place in the cube root of 21952, we will first find the cube root of 21952 with the help of the prime factorization method. To start with, we will do prime factorization of the number 21952. Thus we get,

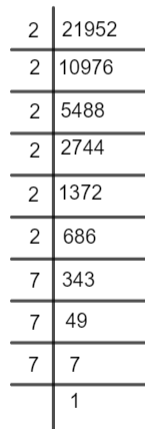
Therefore, we can write 21952 as,
\[21952=2\times 2\times 2\times 2\times 2\times 2\times 7\times 7\times 7\]
Now, we will make groups of three of the same numbers. Thus, we have,
\[21952=\left( 2\times 2\times 2 \right)\times \left( 2\times 2\times 2 \right)\times \left( 7\times 7\times 7 \right)\]
Now, we will select one prime number from each group. Thus, the prime numbers we will get are 2, 2, and 7.
Now, the cube root of the number 21952 will be equal to the product of these numbers which we have selected from each group. Thus, we have,
\[\sqrt[3]{21952}=2\times 2\times 7\]
\[\Rightarrow \sqrt[3]{21952}=28\]
Now, the digit at the units place of the cube root of 21952 is 8.
Hence, option (a) is the right answer.

Note: The method of prime factorization which we have applied while solving the question cannot be used every time. It can be used only when the given number is the cube of an integer. In our case, 21952 is the cube of 28, so we can use this method. We can use another technique. We know that the unit digit of the cube of the unit digit of the cube root would be the unit digit of the given number. For example, here we have. For example, here we have
\[8\times 8\times 8=512\]
\[6\times 6\times 6=216\]
\[4\times 4\times 4=64\]
\[2\times 2\times 2=8\]
From the above, it is clear that 2 is the unit digit of \[{{8}^{3}}\] and the unit digit of 21952 is also 2, so the unit digit of the cube root of 21952 would also be 8.