
What will be the direction of frictional force on block A?
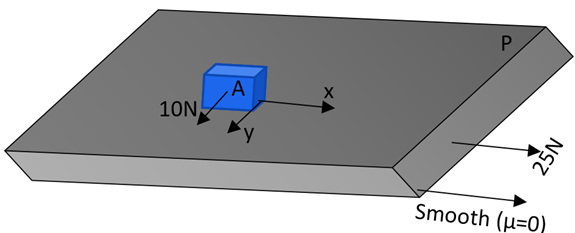
A. Towards positive x-axis
B. Towards negative y-axis
C. At an angle of $45{}^\circ $ from positive x-axis and negative y-axis
D. none of these

Answer
462.6k+ views
Hint: here the maximum friction acting on A will be insufficient in order to make a movement in both x-direction and y-direction. Calculate the frictional force on A in both the directions separately. Then find out the frictional of the platform. From that arrive at the frictional force acting in the y- direction. The total frictional force will be the square root of the sum of the squares of frictional forces in each direction. Find out the tangent of the angle and from this arrive at the value of angle.
Complete answer:
As the maximum friction that can act on A is given as¸
$\left( 0.06 \right)\left( 100 \right)=6N$
Then it will be insufficient to make motion in both x and y directions. Therefore, let friction in x direction be ${{f}_{x}}$ and that in y direction be ${{f}_{y}}$.
Now for the block A in x-direction, only friction acts. This can be written as,
${{f}_{x}}=10{{a}_{Ax}}$
In the y-direction, both the frictional force and external force will be acting. That is,
$10-{{f}_{y}}=10{{a}_{Ay}}$
In the same way, we can find the forces for the platform also. That is,
\[\begin{align}
& 25-{{f}_{x}}=25{{a}_{Bx}} \\
& {{f}_{y}}=25{{a}_{By}} \\
\end{align}\]
Hence the total frictional force can be found as,
\[F=\sqrt{{{f}_{x}}^{2}+{{f}_{y}}^{2}}=\mu N=6\]
And also, as the friction is acting opposite to the relative motion,
\[\tan \theta =\dfrac{{{a}_{Ax}}-{{a}_{Bx}}}{{{a}_{Ay}}-{{a}_{By}}}=\dfrac{{{f}_{x}}}{{{f}_{y}}}\]
When we solve this equation will give that,
\[\tan \theta =1\]
Therefore,
\[\theta =45{}^\circ \]
So, the correct answer is “Option C”.
Note:
Frictional force is a kind of opposing force which is developed by two surfaces when they come in contact and slide against each other. This force will be opposite to the external force acting on the body. The surface texture and the amount of force given are the factors affecting the frictional force.
Complete answer:
As the maximum friction that can act on A is given as¸
$\left( 0.06 \right)\left( 100 \right)=6N$
Then it will be insufficient to make motion in both x and y directions. Therefore, let friction in x direction be ${{f}_{x}}$ and that in y direction be ${{f}_{y}}$.
Now for the block A in x-direction, only friction acts. This can be written as,
${{f}_{x}}=10{{a}_{Ax}}$
In the y-direction, both the frictional force and external force will be acting. That is,
$10-{{f}_{y}}=10{{a}_{Ay}}$
In the same way, we can find the forces for the platform also. That is,
\[\begin{align}
& 25-{{f}_{x}}=25{{a}_{Bx}} \\
& {{f}_{y}}=25{{a}_{By}} \\
\end{align}\]
Hence the total frictional force can be found as,
\[F=\sqrt{{{f}_{x}}^{2}+{{f}_{y}}^{2}}=\mu N=6\]
And also, as the friction is acting opposite to the relative motion,
\[\tan \theta =\dfrac{{{a}_{Ax}}-{{a}_{Bx}}}{{{a}_{Ay}}-{{a}_{By}}}=\dfrac{{{f}_{x}}}{{{f}_{y}}}\]
When we solve this equation will give that,
\[\tan \theta =1\]
Therefore,
\[\theta =45{}^\circ \]
So, the correct answer is “Option C”.
Note:
Frictional force is a kind of opposing force which is developed by two surfaces when they come in contact and slide against each other. This force will be opposite to the external force acting on the body. The surface texture and the amount of force given are the factors affecting the frictional force.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

Trending doubts
10 examples of friction in our daily life

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

State the laws of reflection of light




