
The direction ratios of the diagonal of the cube joining the origin to the opposite corner are (when the three concurrent edges of the cube are coordinate axes.)
a.
b.
c.
d.
Answer
482.4k+ views
1 likes
Hint: Concurrent edges are the set of lines or curves if they all intersect each other at the same point. The point at which all the edges intersect is called the point of concurrency. In the given question the diagonals of the cube join at the origin where origin represents the point of concurrency. Construct a diagram of the cube and let the side of the cube be of
Complete step-by-step answer:
Consider the figure below,
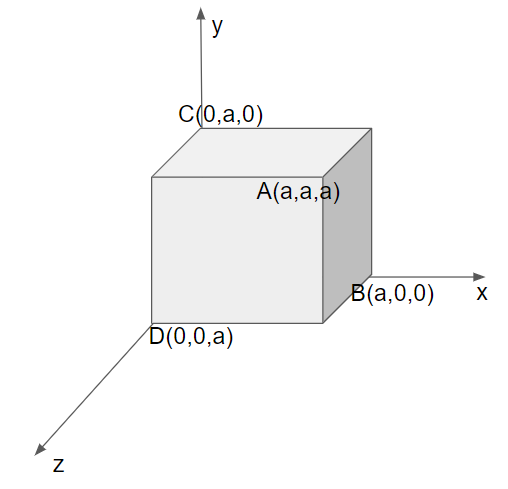
From the figure which represents the cube, we can notice that we have let the side of the cube be
Next, we will find the points at
So, the point which lies in all the planes is
Next, the point which lies on the
Next, the point which lies on the
Further, the point which lies on the
In the question, we are given that the 3 concurrent edges of the cube are coordinate axis which means that
Thus, the point
The opposite corner which is joining the origin is represented by point
Thus, we need to find the direction ratio of the diagonals of the cube joining the origin to the opposite corner.
Direction ratios represent the ratio of each coordinate to the total magnitude
Now, we will find the direction ratio of line
Thus, the direction ratios of diagonal
This gives us,
Therefore,
Hence the direction ratios of the diagonal of the cube joining the origin to the opposite corner are
The option is incorrect as the ratio is
Note: Construct a cube and represent the 3 axes with the points marked on it and the common point which lies in all the 3 planes. The Concurrent edges are the set of lines or curves if they all intersect each other at the same point. The three axes represent the concurrent edges in the figure and at the origin all the 3 edges get intersected.
Complete step-by-step answer:
Consider the figure below,

From the figure which represents the cube, we can notice that we have let the side of the cube be
Next, we will find the points at
So, the point which lies in all the planes is
Next, the point which lies on the
Next, the point which lies on the
Further, the point which lies on the
In the question, we are given that the 3 concurrent edges of the cube are coordinate axis which means that
Thus, the point
The opposite corner which is joining the origin is represented by point
Thus, we need to find the direction ratio of the diagonals of the cube joining the origin to the opposite corner.
Direction ratios represent the ratio of each coordinate to the total magnitude
Now, we will find the direction ratio of line
Thus, the direction ratios of diagonal
This gives us,
Therefore,
Hence the direction ratios of the diagonal of the cube joining the origin to the opposite corner are
The option is incorrect as the ratio is
Note: Construct a cube and represent the 3 axes with the points marked on it and the common point which lies in all the 3 planes. The Concurrent edges are the set of lines or curves if they all intersect each other at the same point. The three axes represent the concurrent edges in the figure and at the origin all the 3 edges get intersected.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




