
The elements in the fifth row of Pascal triangle is
A) 1, 5, 10, 10, 5, 1
B) 1, 6, 15, 20, 15, 6, 1
C) 1, 4, 6, 4, 1
D) 1, 7, 21, 35, 35, 21, 7, 1
Answer
503.4k+ views
Hint: Pascal's triangle determines the coefficients which arise in the binomial expansions.
Pascal’s triangle is the triangular array meaning the arrangement of the binomial coefficient. It is named after the French mathematician Blaise Pascal.
Complete step-by-step answer:
Conventionally the rows of Pascal’s are enumerated starting with the row
Construction of the triangle –
In top most, zeroth row, there is the unique non-zero number i.e. number one.
Each subsequent entry of the row is constructed by adding the number above and to the left with the number above and to the right, treating blank entries as
For example, the initial number in the first row or any other row is
In the Pascal triangle, each entry of the row is the value of the binomial coefficient. So, the simple solution is to construct all row elements up to nth row and add them.
But the approach probably will have O(
Conversely, it can be optimized up to O(
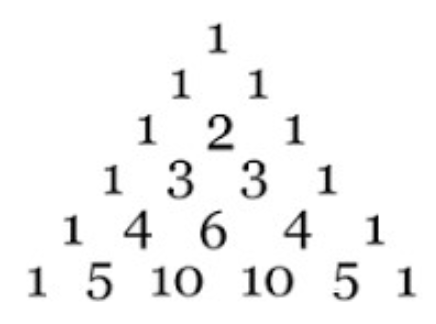
The elements in the fifth row of the Pascal triangle are 1,4,6,4,1.
Note: The sum of the entries in the
Pascal’s triangle is the triangular array meaning the arrangement of the binomial coefficient. It is named after the French mathematician Blaise Pascal.
Complete step-by-step answer:
Conventionally the rows of Pascal’s are enumerated starting with the row
Construction of the triangle –
In top most, zeroth row, there is the unique non-zero number i.e. number one.
Each subsequent entry of the row is constructed by adding the number above and to the left with the number above and to the right, treating blank entries as
For example, the initial number in the first row or any other row is
In the Pascal triangle, each entry of the row is the value of the binomial coefficient. So, the simple solution is to construct all row elements up to nth row and add them.
But the approach probably will have O(
Conversely, it can be optimized up to O(

The elements in the fifth row of the Pascal triangle are 1,4,6,4,1.
Note: The sum of the entries in the
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




