Question

The figure of a centimetre scale below shows a particular position of the Vernier calipers. In this position the value of $x$ shown in the figure is (figure is not to scale).

$A. 0.20\;cm$
$B. 3.65\;cm$
$C. 4.15\;cm$
$D. 0.03\;cm$

Answer 1

Hint: When Vernier scale is used for taking readings, we need to calculate the least count of Vernier and the number of divisions between each measurement. For calculating the value of$x$, we need to find the exact point where any of the markings on the Vernier scale coincides with one of the markings on the main scale.

Complete answer:
Calibration is expressed as the comparison of measurement values generated by a device under test with those of a calibration standard of known accuracy. A Vernier scale is basically a visual aid to take an accurate measurement reading between two graduation markings on a linear scale with the help of mechanical interpolation; thereby increasing the resolution and reducing the measurement uncertainty by using Vernier acuity to reduce possible errors.
The Vernier is a subsidiary measuring scale replacing a single measured-value pointer, and has a total of ten divisions equal in distance to nine divisions on the main scale. The interpolated reading is obtained by carefully observing which of the Vernier scale graduations is coinciding with a graduation on the main scale, which is easier to perceive than the visual estimation between two points. Such an arrangement of Vernier can go to higher resolution by using higher scale ratios, known as the Vernier constant. A Vernier scale can be used on circular or straight scales where a simple linear mechanism is adequate.
Let’s take an example to determine the reading through Vernier caliper:

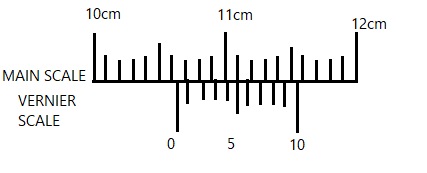
Main scale reading: $10\;cm$(Immediate left of zero)
Vernier scale reading:$0.02\;cm$(Alignment of scale lines)
Measurement reading:$10.02\;cm$
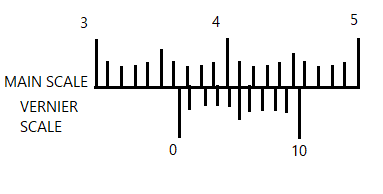
We are given a centimetre scale showing a particular position of Vernier scale. At the ${{5}^{th}}$reading of Vernier’s scale, both readings of the scales coincide. At the ${{4}^{th}}$reading of Vernier’s scale, the length between the two scales is minimum.
The last count of Vernier scale is $0.01\; cm$ So, the difference at ${{3}^{rd}}$reading of Vernier scale is, $2\times 0.01cm=0.002cm$
Difference at ${{2}^{nd}}$reading of Vernier scale is, $3\times 0.01=0.003cm$
Now,
Value of$x$is given as:
$x=0.3cm-3x\text{ scale division of vernier calliper}$
We get,
$x=0.3cm-3\times \dfrac{9}{100}cm$
$x=0.3cm-\dfrac{27}{100}cm$
$x=0.03cm$
The required value of$x$is $0.03\;cm$

Hence, the correct option is D.

Note:
In order to take the measurement readings from Vernier caliper properly, students need to remember the below points:
Consider that Vernier caliper output a measurement of$3.23\;cm$, this means that,
1. The main scale contributes the main number(s), and one decimal place to the reading. For example,$3.2\;cm$, whereby $3$ is the main number and $0.\;2$ is the one decimal place number.
2. The Vernier scale contributes the second decimal place to the measurement reading. For example, $0.03\;cm$.