Answer
456k+ views
Hint: Capacitor is a device which is used to store the charge. The charge stored in the capacitor depends on the potential difference applied across them and also depends on the capacitance of the capacitor. We have different formulas to find out effective capacitance when they are connected in series or parallel.
Formula used:
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
$ {C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}........... $
Complete step-by-step solution:
Generally, when there is more than one capacitor, we can connect them in series or we can connect them in parallel.
In the case of series connection, the current flowing through the circuit will be the same which means that the charge stored on every capacitor will be the same as the charge is nothing but the current flowing in a given time.
In the case of parallel connection, the voltage across all the capacitors will be the same.
In series connection charge will be the same and voltage will be divided across the capacitors whereas in parallel connection voltage will be the same and the charge will be divided across the capacitors.
Effective capacitance for capacitors in series connection is
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
Effective capacitance for capacitors in parallel connection is
${C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}...........$
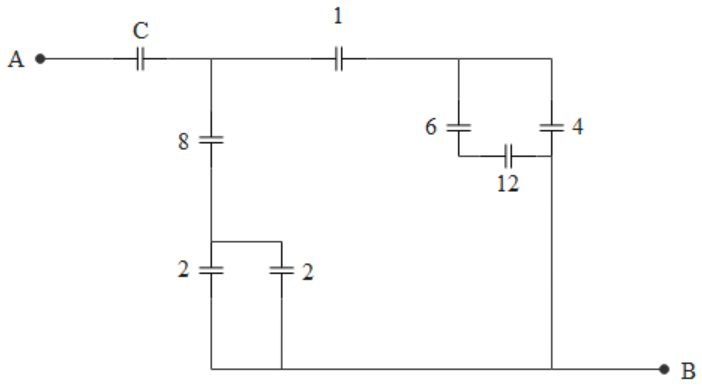
From the given circuit
12 and 6 are in series so effective capacitance will be
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
$\eqalign{
& \Rightarrow \dfrac{1}{{{C_S}}} = \dfrac{1}{{12}} + \dfrac{1}{6} \cr
& \Rightarrow {C_S} = \dfrac{{12 \times 6}}{{12 + 6}} \cr
& \Rightarrow {C_S} = 4 \cr} $
Now this effective 4 and other 4 are in parallel. So the effective capacitance will be
${C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}...........$
$ \Rightarrow {C_P} = 4 + 4 = 8$
Now this 8 and 1 are in series. So the effective capacitance will be
$\eqalign{
& {C_S} = \dfrac{{8 \times 1}}{{8 + 1}} \cr
& \Rightarrow {C_S} = \dfrac{8}{9} \cr} $
Now 2 and 2 at the left bottom are parallel so the effective capacitance will be
${C_P} = 2 + 2 = 4$
This effective 4 is in series with 8. So the effective capacitance will be
$\eqalign{
& {C_S} = \dfrac{{8 \times 4}}{{8 + 4}} \cr
& \Rightarrow {C_S} = \dfrac{{32}}{{12}} \cr
& \Rightarrow {C_S} = \dfrac{8}{3} \cr} $
Now finally this $\dfrac{8}{3}$ and $\dfrac{8}{9}$ are parallel. So the effective capacitance will be
${C_P} = \dfrac{8}{3} + \dfrac{8}{9} = \dfrac{{32}}{9}$
Finally this $\dfrac{{32}}{9}$ and capacitance C will be in series and the effective capacitance must be 1. So C will be
$\eqalign{
& {C_S} = \dfrac{{\dfrac{{32}}{9} \times C}}{{\dfrac{{32}}{9} + C}} \cr
& \Rightarrow 1 = \dfrac{{\dfrac{{32}}{9} \times C}}{{\dfrac{{32}}{9} + C}} \cr
& \Rightarrow \dfrac{{32}}{9} + C = \dfrac{{32}}{9} \times C \cr
& \Rightarrow C = \dfrac{{32}}{{23}}\mu F \cr} $
Hence option A will be the answer.
Note: The formulas which we have for the case of finding effective capacitance of parallel and series connection of capacitors will be exactly opposite to the case of finding effective resistance of parallel and series connection of resistors. If we know the voltage across AB we can find out the total charge passing through the system given as we had got the effective capacitance.
Formula used:
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
$ {C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}........... $
Complete step-by-step solution:
Generally, when there is more than one capacitor, we can connect them in series or we can connect them in parallel.
In the case of series connection, the current flowing through the circuit will be the same which means that the charge stored on every capacitor will be the same as the charge is nothing but the current flowing in a given time.
In the case of parallel connection, the voltage across all the capacitors will be the same.
In series connection charge will be the same and voltage will be divided across the capacitors whereas in parallel connection voltage will be the same and the charge will be divided across the capacitors.
Effective capacitance for capacitors in series connection is
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
Effective capacitance for capacitors in parallel connection is
${C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}...........$

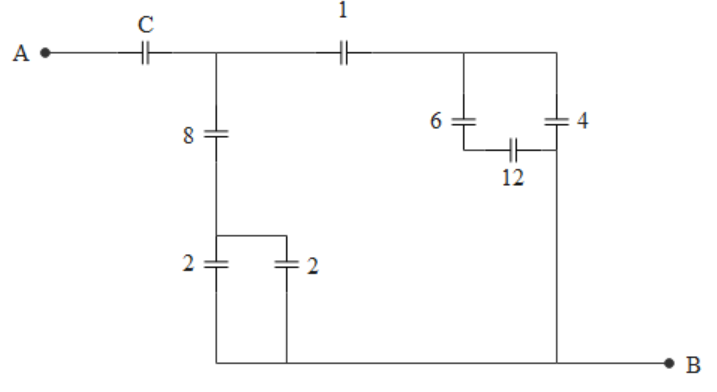
From the given circuit
12 and 6 are in series so effective capacitance will be
$\dfrac{1}{{{C_S}}} = \dfrac{1}{{{C_1}}} + \dfrac{1}{{{C_2}}} + \dfrac{1}{{{C_3}}} + \dfrac{1}{{{C_4}}}{\text{ }}.........$
$\eqalign{
& \Rightarrow \dfrac{1}{{{C_S}}} = \dfrac{1}{{12}} + \dfrac{1}{6} \cr
& \Rightarrow {C_S} = \dfrac{{12 \times 6}}{{12 + 6}} \cr
& \Rightarrow {C_S} = 4 \cr} $
Now this effective 4 and other 4 are in parallel. So the effective capacitance will be
${C_P} = {C_1} + {C_2} + {C_3} + {C_4}{\text{ }}...........$
$ \Rightarrow {C_P} = 4 + 4 = 8$
Now this 8 and 1 are in series. So the effective capacitance will be
$\eqalign{
& {C_S} = \dfrac{{8 \times 1}}{{8 + 1}} \cr
& \Rightarrow {C_S} = \dfrac{8}{9} \cr} $
Now 2 and 2 at the left bottom are parallel so the effective capacitance will be
${C_P} = 2 + 2 = 4$
This effective 4 is in series with 8. So the effective capacitance will be
$\eqalign{
& {C_S} = \dfrac{{8 \times 4}}{{8 + 4}} \cr
& \Rightarrow {C_S} = \dfrac{{32}}{{12}} \cr
& \Rightarrow {C_S} = \dfrac{8}{3} \cr} $
Now finally this $\dfrac{8}{3}$ and $\dfrac{8}{9}$ are parallel. So the effective capacitance will be
${C_P} = \dfrac{8}{3} + \dfrac{8}{9} = \dfrac{{32}}{9}$
Finally this $\dfrac{{32}}{9}$ and capacitance C will be in series and the effective capacitance must be 1. So C will be
$\eqalign{
& {C_S} = \dfrac{{\dfrac{{32}}{9} \times C}}{{\dfrac{{32}}{9} + C}} \cr
& \Rightarrow 1 = \dfrac{{\dfrac{{32}}{9} \times C}}{{\dfrac{{32}}{9} + C}} \cr
& \Rightarrow \dfrac{{32}}{9} + C = \dfrac{{32}}{9} \times C \cr
& \Rightarrow C = \dfrac{{32}}{{23}}\mu F \cr} $
Hence option A will be the answer.
Note: The formulas which we have for the case of finding effective capacitance of parallel and series connection of capacitors will be exactly opposite to the case of finding effective resistance of parallel and series connection of resistors. If we know the voltage across AB we can find out the total charge passing through the system given as we had got the effective capacitance.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

What is the meaning of celestial class 10 social science CBSE

What causes groundwater depletion How can it be re class 10 chemistry CBSE

Under which different types can the following changes class 10 physics CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE