Question

The figure shows a square loop of side 5 cm being moved towards the right at a constant speed of 1cm/sec. The front edge just enters the 20 cm wide magnetic field at $ t = 0 $ . Find the induced emf in the loop at a) $ t = 2s $ , b) $ t = 10s $ , c) $ t = 22s $ , and d) $ t = 30s $ . Find the total heat produced during the interval 0 to 30 s if the resistance of the loop is $ 4.5\,m\Omega $ .

Answer 1

Hint When a loop enters the magnetic field, EMF will be induced in the loop due to Faraday’s law of electromagnetic induction. We need to calculate the distance travelled by the coil as a function of time. The area of the coil inside the loop when it is entering and exiting will contribute to inducing EMF in the loop
Formula used: In the solution we will be using the following formula,
Faraday’s law of electromagnetic induction: $ \phi = B.A $ where $ \phi $ is the flux passing through the loop placed in a magnetic field $ B $ and area $ A $
 $ \Rightarrow H = {I^2}Rt $ where $ H $ is the heat generated when $ I $ is the current in the circuit, $ R $ is the resistance, and $ t $ is the time duration of the flow of current.

Complete step by step answer
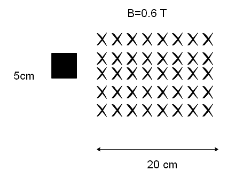
When the loop starts entering the region of the magnetic field, EMF will be induced in the loop due to Faraday's law of electromagnetic induction. Since we want to find out the area of the square loop inside the magnetic field at different times, let us use the relation between distance and velocity to do so. The flux in the square loop can be calculated as
 $ \Rightarrow \phi = B.A $
The EMF induced in the loop will be
 $ \Rightarrow E = \dfrac{d}{{dt}}(B.A) $
Since the magnetic field is constant with respect to time, we can bring out the magnetic field term out of the derivative. Let $ l $ be the length of the side of the loop that is entering the region of the magnetic field and hence has a variable length and $ b $ is its breadth which is constant.
 $ \Rightarrow E = B.\dfrac{d}{{dt}}(l \times b) $
Since the breadth is also constant with time, we can also bring it out of the derivative and substituting $ \dfrac{{dl}}{{dt}} = v $ , we get
 $ \Rightarrow E = Bvb $
a) At $ t = 2s $ ,
The EMF induced at this point will be ( $ v = 0.01\,m/s $ and $ b = 0.05\,m $ )
 $ \Rightarrow E = 0.6 \times 0.01 \times 0.05 $
 $ \Rightarrow E = 3 \times {10^{ - 4}}\,V $
The current in the circuit can be calculated using ohm’s law as
 $ \Rightarrow I = \dfrac{E}{R} $
 $ \Rightarrow I = \dfrac{{3 \times {{10}^{ - 4}}\,}}{{4.5 \times {{10}^{ - 3}}}} = 6.7 \times {10^{ - 2}}A $
b) At $ t = 10s $ , the entire loop will be inside the region of the magnetic field and hence there will be no change in the area of the loop inside the magnetic field and the EMF induced will be zero.
c) At $ t = 22s $ , some part of the square loop will have started to exit the magnetic field and EMF will be induced again as ( $ v = 0.01\,m/s $ and $ b = 0.05\,m $ )
 $ \Rightarrow E = 0.6 \times 0.01 \times 0.05 $
 $ \Rightarrow E = 3 \times {10^{ - 4}}\,V $
The current in the circuit can be calculated using ohm’s law as
 $ \Rightarrow I = \dfrac{E}{R} $
 $ \Rightarrow I = \dfrac{{3 \times {{10}^{ - 4}}\,}}{{4.5 \times {{10}^{ - 3}}}} = 6.7 \times {10^{ - 2}}A $
d) At $ t = 30s $ , the entire loop will be outside the region of the magnetic field so there will be no change in the area inside the magnetic field and hence the EMF generated will be zero.
To find the heat produced in the loop, we know that heat will only be produced in the regions where EMF is generated in the loop which are intervals where the area of the loop inside the magnetic field changes with time i.e. 0-5 s and 20-25 s
The heat generated due to resistance can hence be calculated using the formula
 $ \Rightarrow H = {I^2}Rt $ where $ H $ is the heat generated when $ I $ is the current in the circuit, $ R $ is the resistance and $ t $ is the time duration of the flow of current which is 5 s in our case as the loop is completely inside the region of the magnetic field in 5 s.
Since in both the time frames of interest, the current is the same and so is the time duration, the total heat generated will be twice the heat generated in one duration. So substituting $ I = 6.7 \times {10^{ - 2}} $ and $ t = 5\,s $ , we get
 $ \Rightarrow H = 2 \times {\left( {6.7 \times {{10}^{ - 2}}} \right)^2} \times 4.5 \times {10^{ - 3}} \times 5 $
 $ \Rightarrow H = 2 \times {10^{ - 4}}\,{\text{Joules}} $ .

Note
We must be careful in only taking the area of the loop that is inside the region of the magnetic field when it is entering the region and the area of the loop that is outside the magnetic field when it is exiting the region since these regions will experience a change in magnetic flux and hence generating an EMF. Here we have to assume that the coil is constantly being pushed to make it move with a constant velocity otherwise the loop will stop moving due to Lenz law once it starts entering the magnetic field.