
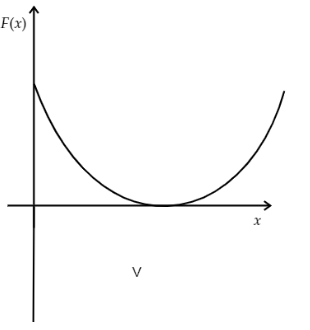
The first graph shows the potential energy U(x) for a particle moving on the x-axis.Which of the following graphs will correctly give the force

(I)
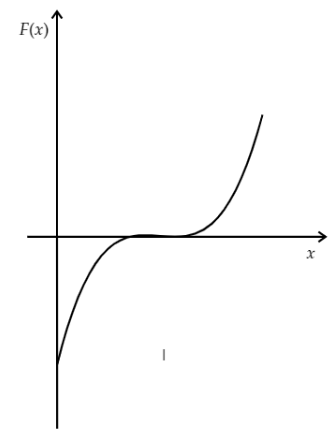
(II)
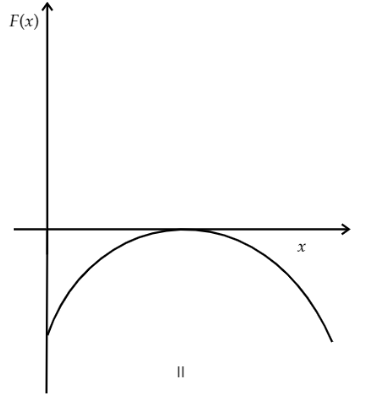
(III)
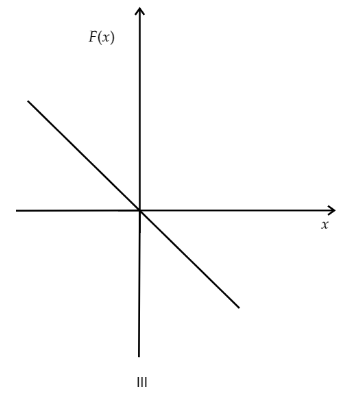
(IV)
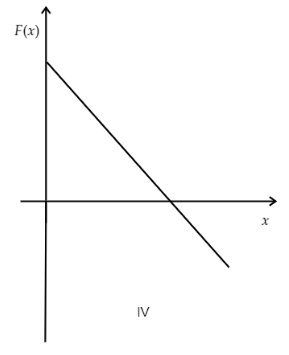
(V)
A. I
B. II
C. III
D. IV
E. V






Answer
422.4k+ views
Hint:Use the relation between the potential energy of a system with the force and displacement to recognize the correct graph. Potential energy is a type of energy of a system which is stored in the field and only depends on the position of the system, it is independent of momentum of the system.
Formula used:
The force of a system is related to potential energy as,
Where,
Complete step by step answer:
We know that the potential energy of a 1D system is generated due to the force
Now, here we can see that the graph is a parabola and it is of the form,
Now, if we partially differentiate w.r.t x we will have,
So, the force exerted on the particle will be,
Hence, we can see that it is a straight line with slope
So, the force will also have some value of
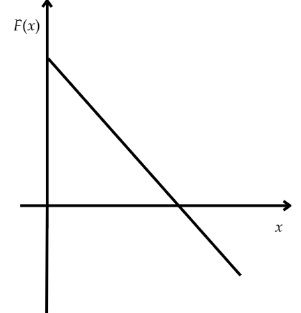
So, we can see that this only matches with the curve number (IV).
Hence, option D is the correct answer.
Note:The force law for a potential of a system is nothing but the slope of the curve. So, if we can know the nature of the curve we can easily find the slope at each point of the curve by differentiating at each point or the expression. Curve (III) and (IV) can be confusing since both of them are straight lines. But we can easily differentiate between them using the boundary conditions at
Formula used:
The force of a system is related to potential energy as,
Where,
Complete step by step answer:
We know that the potential energy of a 1D system is generated due to the force
Now, here we can see that the graph is a parabola and it is of the form,
Now, if we partially differentiate w.r.t x we will have,
So, the force exerted on the particle will be,
Hence, we can see that it is a straight line with slope
So, the force will also have some value of

So, we can see that this only matches with the curve number (IV).
Hence, option D is the correct answer.
Note:The force law for a potential of a system is nothing but the slope of the curve. So, if we can know the nature of the curve we can easily find the slope at each point of the curve by differentiating at each point or the expression. Curve (III) and (IV) can be confusing since both of them are straight lines. But we can easily differentiate between them using the boundary conditions at
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




