
The focal distance of a point on the parabola
A). (9, 6)
B). (25, 10)
C). (25, -10)
D). None of these
Answer
502.8k+ views
Hint: Before attempting this question one must have prior knowledge of parabola, the equation which represents the parabola is
Complete step-by-step solution -
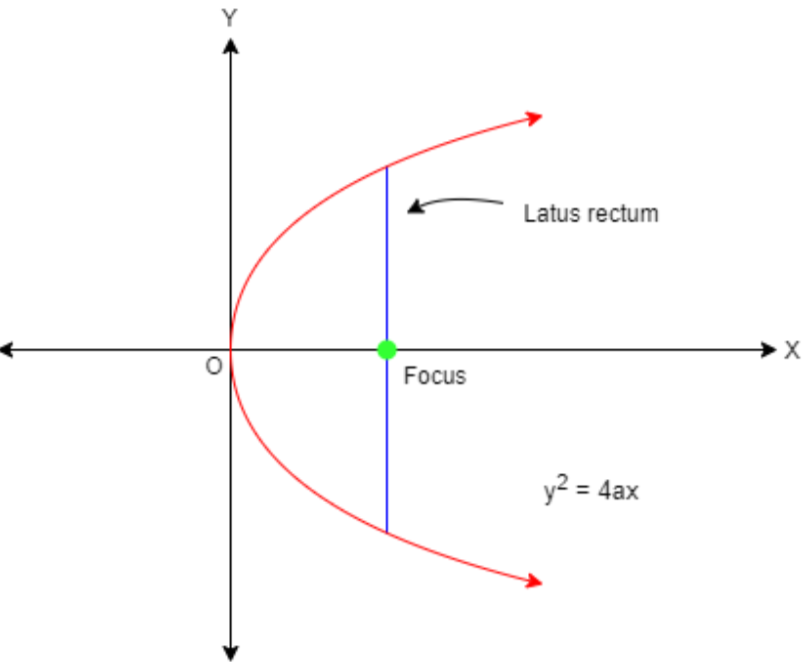
According to the given information we have a parabola
We know that the general equation of parabola is
Taking the given equation of parabola i.e.
Comparing the general equation of parabola with the given equation of parabola we get
So the coordinates of focus of parabola will be (1, 0)
We have focus distance = 10
So focal distance = 10 =
Squaring both sides we get
Substituting the value of
We get
By the method of splitting the middle term method
We get
So
Since x can’t be negative
Now substituting the value of x in equation 1
For x = 9
So
Therefore the coordinates are (9, 6)
Hence option A is the correct option.
Note: In the above solution we came across the terms parabola and focal distance which can be explained as a curve which consists of a set of all points that exist at equal distance from a fixed point (focus) this curve is named as a parabola. The distance from the vertex to focus which is measured along the symmetry of the axis is called focal distance.
Complete step-by-step solution -

According to the given information we have a parabola
We know that the general equation of parabola is
Taking the given equation of parabola i.e.
Comparing the general equation of parabola with the given equation of parabola we get
So the coordinates of focus of parabola will be (1, 0)
We have focus distance = 10
So focal distance = 10 =
Squaring both sides we get
Substituting the value of
We get
By the method of splitting the middle term method
We get
So
Since x can’t be negative
Now substituting the value of x in equation 1
For x = 9
So
Therefore the coordinates are (9, 6)
Hence option A is the correct option.
Note: In the above solution we came across the terms parabola and focal distance which can be explained as a curve which consists of a set of all points that exist at equal distance from a fixed point (focus) this curve is named as a parabola. The distance from the vertex to focus which is measured along the symmetry of the axis is called focal distance.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




