
The graph between the square root of the frequency of a specific line of characteristic spectrum of X-ray and the atomic number of the target will be
a)
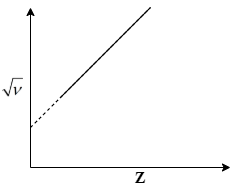
b)

c)
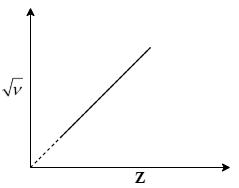
d)
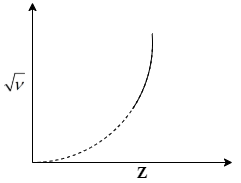




Answer
495k+ views
2 likes
Hint:It has been observed that the frequency of the characteristic spectrum of X-ray and the atomic number of the target is dependent in such a way that the frequency is proportional to the square of the atomic number with an offset value.
Formula used:
Relationship between the frequency of characteristic spectrum of X-ray and the atomic number is given by:
Where,
Z denotes the atomic number of the target atom,
a is the proportionality constant and a is positive,
b is offset constant and it’s positive also.
Complete step by step answer:
From eq.(1) we get the relationship between
Now, compare this eq.(2) with the equation of a straight line i.e.
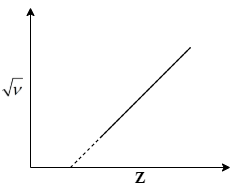
Where, square roots of frequency values are given at y axis and the atomic number Z is given in the x-axis.
Correct answer:
The correct graph is given in option (b).
Note: From the given relation between frequency of characteristic spectrum and atomic number in eq.(1) a student can easily determine that the relationship between
Formula used:
Relationship between the frequency of characteristic spectrum of X-ray and the atomic number is given by:
Where,
Z denotes the atomic number of the target atom,
a is the proportionality constant and a is positive,
b is offset constant and it’s positive also.
Complete step by step answer:
From eq.(1) we get the relationship between
Now, compare this eq.(2) with the equation of a straight line i.e.

Where, square roots of frequency values are given at y axis and the atomic number Z is given in the x-axis.
Correct answer:
The correct graph is given in option (b).
Note: From the given relation between frequency of characteristic spectrum and atomic number in eq.(1) a student can easily determine that the relationship between
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




