Question

The gravitational force of each planet in our solar system is different. The diagram below shows four planets listed in order from least amount of relative gravity to greatest amount of relative gravity. A person would weigh the most on which planet.

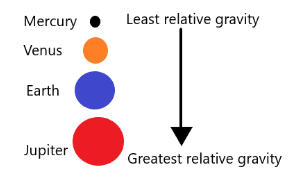
(A) Mercury
(B) Venus
(C) Earth
(D) Jupiter

Answer 1

Hint: The weight of a body is a result of the gravitational force between the body and the planet. The gravitational force a planet can exert is directly related to the density, hence relative gravity (also called relative density or specific gravity in some text) of the planet.
Formula used: In this solution we will be using the following formulae;
 $\Rightarrow W = mg $ where $ W $ is the weight of a body in a gravitational field of a planet, $ m $ is the mass of the body and $ g $ is the acceleration due to gravity of the planet.
 $\Rightarrow F = G\dfrac{{mM}}{{{r^2}}} $ where $ F $ is the gravitational force between the planet and a body at a distance $ r $ from the centre of the planet, $ m $ is the mass of the object and $ M $ is the mass of the planet. $ G $ is the gravitational constant.

Complete step by step solution:
According to Newton’s universal law of gravitation, two bodies such as an object and a planet, exert a force on each other that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them. Mathematically represented as
$F = G\dfrac{{mM}}{{{r^2}}} $ where $ m $ is the mass of the object and $ M $ is the mass of the planet, while $ r $ is the distance between the planet and the object, and $ G $ is the gravitational constant.
Now, the weight of a body, given as $ W = mg $ , $ g $ is the acceleration due to gravity of the planet, is an effect of the gravitational force between the planet and the body. Hence, we can say
 $\Rightarrow W = mg = G\dfrac{{mM}}{{{r^2}}} $
 $\Rightarrow mg = G\dfrac{{mM}}{{{r^2}}} $
Hence by cancelling $ m $ we have
 $\Rightarrow g = G\dfrac{M}{{{r^2}}} $
Thus, by investigating the acceleration due to gravity of the planet we can demonstrate on which planet the person is heavier.
The density of a substance is defined as $ \rho = \dfrac{M}{V} $ where $ V $ is volume and $ M $ is mass.
Multiplying both the numerator and the denominator of the RHS of $ g = G\dfrac{M}{{{r^2}}} $ by $ \dfrac{4}{3}\pi r $ we have
 $\Rightarrow g = G\dfrac{{\dfrac{4}{3}\pi Mr}}{{\dfrac{4}{3}\pi {r^3}}} $ . If the person is on the surface of the planet then $ r = R $ where $ R $ is the radius of the planet. Hence
 $\Rightarrow g = G\dfrac{{\dfrac{4}{3}\pi MR}}{{\dfrac{4}{3}\pi {R^3}}} = \dfrac{4}{3}\pi GR\dfrac{M}{V} = \dfrac{4}{3}\pi GR\rho $ .
 $ \dfrac{4}{3}\pi {R^3} $ is the volume of a sphere.
Density $ \rho = SG \times {\rho _r} $ where $ {\rho _r} $ is the density of the reference (usually water) and SG is the specific gravity. Hence
 $\Rightarrow g = \dfrac{4}{3}\pi GR{\rho _r}SG $ . This implies that an increase in SG increases the acceleration due to gravity of the planet hence the force of gravity, and thus the weight of the person.
Jupiter has the greatest specific gravity in the option.
Hence, the correct option is D.

Note:
However, it should be noted that an increase in mass of the planet does not necessarily increase the specific gravity of the planet. For example, if the mass of the planet increases and its size increases by the same ratio, the specific gravity actually reduces.