Question

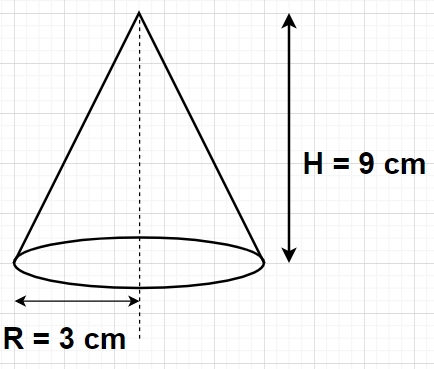
The height of a cone and the radius of its base are respectively 9 and 3 cm. The cone is cut by a plane parallel to its base so as to divide it into two parts. The volume of frustum of cone is 44 ${\text{c}}{{\text{m}}^3}$, then what is the radius of upper circular of frustum (Use $\pi = \dfrac{{22}}{7}$) ?
\[
  {\text{A}}{\text{. }}\sqrt[3]{{12}}{\text{ cm}} \\
  {\text{B}}{\text{. }}\sqrt[3]{{13}}{\text{ cm}} \\
  {\text{C}}{\text{. }}\sqrt[3]{6}{\text{ cm}} \\
  {\text{D}}{\text{. }}\sqrt[3]{{20}}{\text{ cm}} \\
 \]

Answer 1

Hint- Here, we will proceed by developing a relation between the height and the radius of the smaller cone obtained after the original cone is cut by a plane. This is achieved with the help of the concept of similar triangles.

Complete step by step answer:
Given, Height of the cone, H = 9 cm
Radius of the base of the cone, R = 3 cm
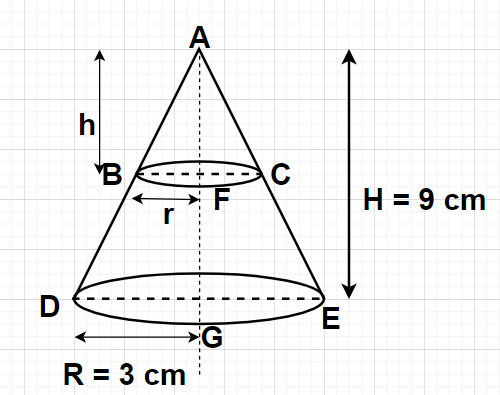
Now, this cone is cut by a plane parallel to its base so as to divide this original cone into a smaller cone and a frustum. Let the radius of the smaller (upper) circular end of the frustum be r cm and the height of the smaller cone obtained be h cm.
The radius of the larger (lower) circular end of frustum is equal to the radius of the original cone i.e., R = 3 cm
Height of frustum = (H – h) cm = (9-h) cm
In triangles ABC and ADE,
$\angle {\text{A}} = \angle {\text{A}}$ [Common angle in both the triangles]
$\angle {\text{ABC}} = \angle {\text{ADE}}$ [Corresponding angles (because BC is parallel to DE) are equal in measure]
$\angle {\text{ACB}} = \angle {\text{AED}}$ [Corresponding angles (because BC is parallel to DE) are equal in measure]
By AAA (Angle-Angle-Angle) similarity rule, we can say that the triangles ABC and ADE are similar to each other i.e., $\vartriangle {\text{ABC}} \sim \vartriangle {\text{ADE}}$.
For any two similar triangles, the ratio of their corresponding dimensions are always equal.
Using the above concept for the two similar triangles i.e., ABC and ADE, we have
$
   \Rightarrow \dfrac{{{\text{AF}}}}{{{\text{AG}}}} = \dfrac{{{\text{BF}}}}{{{\text{DG}}}} \\
   \Rightarrow \dfrac{h}{H} = \dfrac{r}{R} \\
   \Rightarrow \dfrac{h}{9} = \dfrac{r}{3} \\
   \Rightarrow h = \dfrac{{9r}}{3} \\
   \Rightarrow h = 3r \\
 $
Since, volume of any cone is given by
Volume of the cone = $\dfrac{1}{3}\pi {\left( {{\text{Base radius}}} \right)^2} \times \left( {{\text{Height}}} \right)$
As, we know that volume of the frustum will be given by
Volume of frustum = Volume of original cone – Volume of the smaller cone
$ \Rightarrow $ Volume of frustum = $\dfrac{1}{3}\pi {\left( R \right)^2}\left( H \right) - \dfrac{1}{3}\pi {\left( r \right)^2}\left( h \right)$
By putting R = 3 cm, H = 9 cm, h = 3r and $\pi = \dfrac{{22}}{7}$ in the above equation, we get
 $ \Rightarrow $ Volume of frustum = $\dfrac{1}{3}\pi {\left( 3 \right)^2}\left( 9 \right) - \dfrac{1}{3}\pi {\left( r \right)^2}\left( {3r} \right) = \left( {\dfrac{{22}}{7}} \right)\left( 3 \right)\left( 9 \right) - \left( {\dfrac{{22}}{7}} \right){\left( r \right)^2}\left( r \right) = \left( {\dfrac{{22}}{7}} \right)\left( {27 - {r^3}} \right)$
It is given that the volume of frustum is 44 ${\text{c}}{{\text{m}}^3}$
$
   \Rightarrow 44 = \left( {\dfrac{{22}}{7}} \right)\left( {27 - {r^3}} \right) \\
   \Rightarrow 27 - {r^3} = \dfrac{{44 \times 7}}{{22}} = 2 \times 7 = 14 \\
   \Rightarrow {r^3} = 27 - 14 = 13 \\
   \Rightarrow r = \sqrt[3]{{13}}{\text{ cm}} \\
 $
Therefore, the radius of the upper circular of frustum is $\sqrt[3]{{13}}{\text{ }}$cm.

Hence, option B is correct.

Note- In this particular problem, triangles ABC and ADE are similar triangles so, we can write the ratios of the corresponding sides will be equal i.e.,$\dfrac{{{\text{AB}}}}{{{\text{AD}}}} = \dfrac{{{\text{BC}}}}{{{\text{DE}}}} = \dfrac{{{\text{AC}}}}{{{\text{AE}}}}$. In case of similar triangles, the ratio of the altitudes will be equal to the ratio of the corresponding sides i.e., $\dfrac{{{\text{BC}}}}{{{\text{DE}}}} = \dfrac{{{\text{AF}}}}{{{\text{AG}}}} \Rightarrow \dfrac{{{\text{2BF}}}}{{{\text{2DG}}}} = \dfrac{{{\text{AF}}}}{{{\text{AG}}}} \Rightarrow \dfrac{{{\text{BF}}}}{{{\text{DG}}}} = \dfrac{{{\text{AF}}}}{{{\text{AG}}}}$.