Question

The height of a parallelogram is one third of its base. If the area of the parallelogram is \[192c{m^2}\] , find its height and base.

Answer 1

Hint: Here in this question, we have to find the height and base of the parallelogram. To solve this by using a formula of area of parallelogram i.e., \[Area = base \times height\] . On substituting the data given in question and by further simplification, we get the required value of height and base.

Complete step-by-step answer:

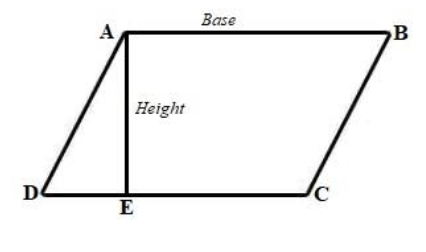
A parallelogram is a quadrilateral with two pairs of parallel sides. The opposite sides of a parallelogram are equal in length, and the opposite angles are equal in measure.
Let a parallelogram \[ABCD\] , sides \[AB\parallel CD\] and \[AD\parallel BC\] . Draw a line AE perpendicular to the line DC i.e., \[AE \bot DC\] where \[AE\] be height if the parallelogram and \[AC\] or \[AB\] be the base of the parallelogram \[ABCD\] .
Let us take the base of the parallelogram be \[x\] i.e., \[Base = xcm\] .
Given,
The height of a parallelogram is one third of its base
 \[ \Rightarrow height = \dfrac{1}{3} \times base\]
 \[ \Rightarrow height = \dfrac{1}{3} \times x\]
 \[ \Rightarrow height = \dfrac{x}{3}cm\]
Now, consider the formula of area of a parallelogram:
 \[ \Rightarrow Area = base \times height\]
 \[ \Rightarrow Area = x \times \dfrac{x}{3}\]
 \[ \Rightarrow Area = \dfrac{{{x^2}}}{3}\]
Given the area of parallelogram is \[192c{m^2}\] , then
 \[ \Rightarrow 192 = \dfrac{{{x^2}}}{3}\]
Multiply 3 on both sides, then
 \[ \Rightarrow 576 = {x^2}\]
Or
 \[ \Rightarrow {x^2} = 576\]
Take square root on both sides, then
 \[ \Rightarrow x = \pm \sqrt {576} \]
As we know 576 is a square number of 24, then
 \[ \Rightarrow x = \pm 24\]
Remember, the measurements of any shape should be in positive, then
 \[ \Rightarrow x = 24\]
Hence, the base of parallelogram is \[x = 24\] cm and
The height is \[height = \dfrac{x}{3}cm\]
 \[ \Rightarrow height = \dfrac{{24}}{3}\]
 \[ \Rightarrow height = 8\] cm.

Note: While determining the height and base we have used the formula of area. The formula is \[Area = base \times height\] We can alter the formula depending upon the which one we want and remember the unit for the area will be the square of the unit of the length of side of a parallelogram. We should not forget to write the unit.