
The internal bisector of an angle of a triangle divides the opposite side internally in the ratio of corresponding sides containing angles. Prove it.
Answer
525.6k+ views
Hint: To solve this problem we need to have a basic knowledge about the properties of triangles and Thales theorem and a basic idea about the construction part which helps us to solve the problem in a simple way by using the required theorems.
Complete step-by-step answer:
Let us consider a triangle ABC.
Here AD is the internal bisector of
According to the question given
We have to prove that
Now here let us do a simple construction which helps to prove the above condition.
Construction: Draw
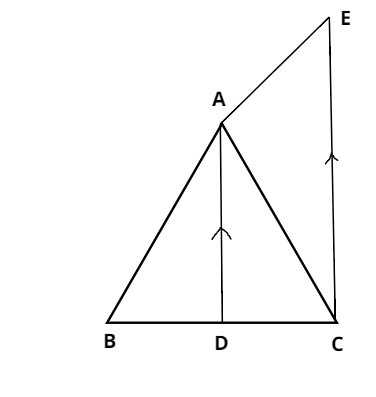
After the construction part is done, we can say that
Since AD is the angle bisector of
Therefore we can say that
So from (1) (2) and (3) we say that
Now from triangle
Again now from triangle BCE
Hence we have proved that the internal bisector of an angle of a triangle divides the opposite side internally in the ratio of corresponding sides containing angles.
NOTE: This question is generally a theorem type question that we have to prove by considering the required concepts. For this type of proof question we have to do required construction to prove the condition. We generally take other theorems to prove the given theorem, in this theorem we have used Thales theorem and some required properties of triangles.
Complete step-by-step answer:
Let us consider a triangle ABC.
Here AD is the internal bisector of
According to the question given
We have to prove that
Now here let us do a simple construction which helps to prove the above condition.
Construction: Draw

After the construction part is done, we can say that
Since AD is the angle bisector of
Therefore we can say that
So from (1) (2) and (3) we say that
Now from triangle
Again now from triangle BCE
Hence we have proved that the internal bisector of an angle of a triangle divides the opposite side internally in the ratio of corresponding sides containing angles.
NOTE: This question is generally a theorem type question that we have to prove by considering the required concepts. For this type of proof question we have to do required construction to prove the condition. We generally take other theorems to prove the given theorem, in this theorem we have used Thales theorem and some required properties of triangles.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




