
The length of the chord of the circle
(A)
(B)
(C)
(D) None of these
Answer
489.9k+ views
Hint: For answering this question we will put
Complete step-by-step solution:
We have the equation of the circle from the question as
The equation of the chord of this circle along the y-axis is given by substituting
After substituting we will have
This is a quadratic equation which can be simply written as
Hence, 3 and 4 are the factors of this equation. So we can say that
Now the length of the chord can be derived by finding the distance between the two endpoints.
From the basic concept we know that for any two points lying on the y-axis the distance between them is given as
Hence the length of the chord of the circle
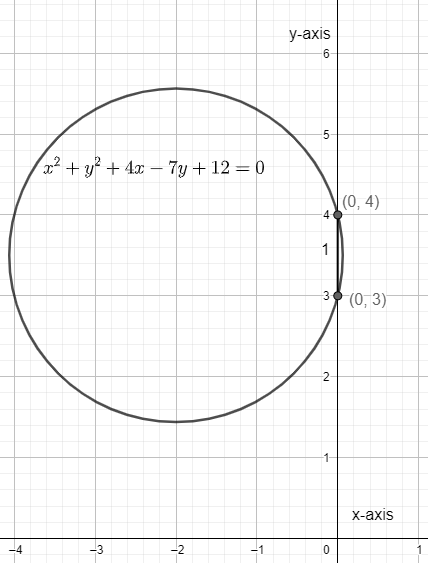
Hence, option A is correct.
Note: While answering this type of question we should take care while obtaining the endpoints of the chord by deriving the roots of the equation of the chord if in case we made a mistake and obtained the roots as follows
Complete step-by-step solution:
We have the equation of the circle from the question as
The equation of the chord of this circle along the y-axis is given by substituting
After substituting we will have
This is a quadratic equation which can be simply written as
Hence, 3 and 4 are the factors of this equation. So we can say that
Now the length of the chord can be derived by finding the distance between the two endpoints.
From the basic concept we know that for any two points lying on the y-axis the distance between them is given as
Hence the length of the chord of the circle

Hence, option A is correct.
Note: While answering this type of question we should take care while obtaining the endpoints of the chord by deriving the roots of the equation of the chord if in case we made a mistake and obtained the roots as follows
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




