Question

The length of the normal chord to the parabola \[{y^2} = 4x\] which subtends a right angle at the vertex is_____
A. \[6\sqrt 3 \]
B. \[3\sqrt 3 \]
C. \[2\]
D. \[1\]

Answer 1

Hint: A line segment passing through any two points on the parabola is known as a chord, and the chord which is perpendicular to the tangent of the parabola at the point of intersection is known as a normal chord.

Complete step by step answer:

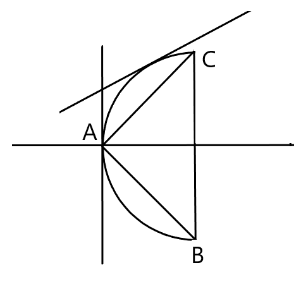
We are assuming CB as the chord to the parabola \[{y^2} = 4x\] where \[a = 1\]
Let \[\left( {a{t^2},2a{t_1}} \right)\]\[\left( {a{t_2}^2,2a{t_2}} \right)\] be the coordinates of C and B respectively. So, the equation becomes at point C,
\[y - 2{t^1} = \dfrac{{ - 2t}}{2}\left( {x - {t^2}} \right)\]
\[\Rightarrow y - 2{t^1} = - t\left( {x - {t^2}} \right)\].................(equation 1)
Therefore, (slope of CA) (slope of AB)\[ = - 1\]

As its given in the question, that the normal chord subtends a right angle at the vector
\[\left( {\dfrac{{\left( {2t - 0} \right)}}{{\left( {{t^2} - 0} \right)}}} \right)\left( {\dfrac{{\left( {2{t_2} - 0} \right)}}{{\left( {t_2^2 - 0} \right)}}} \right) = - 1\]
\[\Rightarrow \left( {\dfrac{{2t}}{{{t^2}}}} \right)\left( {\dfrac{{2{t_2}}}{{t_2^2}}} \right) = - 1\]
\[\Rightarrow {t_1}{t_2} = - 4\]..................( equation 2)
From equation 2,
\[ - {t_1}\left( {{t_2} + {t_1}} \right) = 2\]
\[\Rightarrow 4 - t_1^2 = 2\]....................(equation 3)
\[\Rightarrow t_1^{} = \sqrt 2 \]

Substituting \[{t_1}\] in 3 equation = \[{t_2} = \dfrac{{ - 4}}{{\sqrt 2 }} \]
\[\Rightarrow \dfrac{{ - 4\sqrt 2 }}{2} \Rightarrow - 2\sqrt 2 \]
Coordinates of C= \[\left( {2,2\sqrt 2 } \right)\]
Coordinates of B=\[\left( {8, - 4\sqrt 2 } \right)\]
Therefore the length of the normal chord is, here we are using the distance formula we can get the distance between the two coordinates C and B respectively.
\[\sqrt {{{\left( {8 - 2} \right)}^2} + {{\left( { - 4\sqrt 2 - 2\sqrt 2 } \right)}^2}} \\
\Rightarrow \sqrt {{6^2} + {{\left( { - 6\sqrt 2 } \right)}^2}} \\
\Rightarrow \sqrt {108} \\
\therefore 6\sqrt 3 \]
In the end we came to a conclusion that the answer is \[6\sqrt 3 \] units.

So, option A is the correct option.

Note:In the above solution, distance formula has been used to calculate the distance between two coordinates given. It is an application of the Pythagorean theorem.Interestingly, a lot of people don't actually memorize this formula. Instead, they set up a right triangle, and use the Pythagorean theorem whenever they want to find the distance between two points.