
The locus of the foot of perpendicular drawn from the centre of the ellipse
(a)
(b)
(c)
(d)
Answer
506.4k+ views
1 likes
Hint: First, before proceeding for this, by rearranging the given ellipse equation by dividing both sides by 6 to get standard form as
Complete step-by-step answer:
In this question, we are supposed to find the locus of the foot of perpendicular drawn from the centre of the ellipse
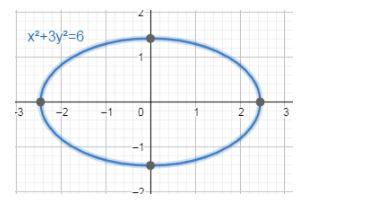
So, before proceeding for this, by rearranging the given ellipse equation by dividing both sides by 6, we get:
Now, by comparing the above equation with the standard form of the ellipse as
Then, we must know the formula for the tangent of the ellipse
Then, by substituting the values we calculated above as
Now, we need to find the equation of the line through the point (0, 0) and perpendicular to 1 is given by:
Then, by substituting the value of m calculated above in the tangent equation, we get:
Now, we need to solve the above equation until we get an expression similar to any of the options given as:
So, the locus of the foot of perpendicular drawn from the centre of the ellipse
So, the correct answer is “Option (c)”.
Note: Now, to solve these type of the questions we need to know some of the basic fundamentals of the equation of the line given by
Complete step-by-step answer:
In this question, we are supposed to find the locus of the foot of perpendicular drawn from the centre of the ellipse

So, before proceeding for this, by rearranging the given ellipse equation by dividing both sides by 6, we get:
Now, by comparing the above equation with the standard form of the ellipse as
Then, we must know the formula for the tangent of the ellipse
Then, by substituting the values we calculated above as
Now, we need to find the equation of the line through the point (0, 0) and perpendicular to 1 is given by:
Then, by substituting the value of m calculated above in the tangent equation, we get:
Now, we need to solve the above equation until we get an expression similar to any of the options given as:
So, the locus of the foot of perpendicular drawn from the centre of the ellipse
So, the correct answer is “Option (c)”.
Note: Now, to solve these type of the questions we need to know some of the basic fundamentals of the equation of the line given by
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




