
The locus of the mid-point of those chord of the circle
(a)
(b)
(c)
(d)
Answer
499.8k+ views
Hint: This question is based on concept of locus and property of chord of circle. First of all, we assume the coordinate of the midpoint of the chord is
(i) Mid-point Theorem: If
(ii) Perpendicular formula: If two lines of slope
Complete step-by-step answer:
Now, getting started with the solution, let’s write the given data.
Given equation of circle is
Centre is
So, the circle can be represented as –
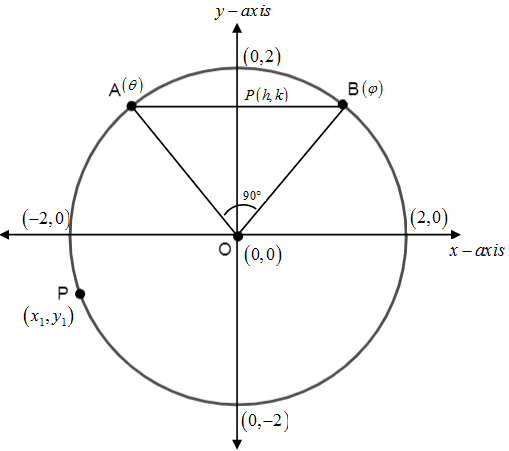
Let
As we know that, slope of line joining two points
So, slope of line
And, slope of line
Now, as we know that multiplication of two perpendicular lines of slope
According to the question,
Now as we know that midpoint
Now let us assume midpoint of chord
Then,
Now by adding squares of equation (iii) and (iv), we get
Now by equation (ii),
Now by replacing
So, the locus of the midpoint of chord is a circle,
So, the correct answer is “Option A”.
Note: (i) In this type of question while solving equations, we should try to eliminate all other variables except
(ii) In this question, we get an equation
If we consider formula:
We can use this relation also to solve the equation at last.
(iii) Here, students should take care while squaring and adding the two equations that there should not be any calculation mistakes, otherwise the whole question will be wrong.
(i) Mid-point Theorem: If
(ii) Perpendicular formula: If two lines of slope
Complete step-by-step answer:
Now, getting started with the solution, let’s write the given data.
Given equation of circle is
Centre is
So, the circle can be represented as –

Let
As we know that, slope of line joining two points
So, slope of line
And, slope of line
Now, as we know that multiplication of two perpendicular lines of slope
According to the question,
Now as we know that midpoint
Now let us assume midpoint of chord
Then,
Now by adding squares of equation (iii) and (iv), we get
Now by equation (ii),
Now by replacing
So, the locus of the midpoint of chord is a circle,
So, the correct answer is “Option A”.
Note: (i) In this type of question while solving equations, we should try to eliminate all other variables except
(ii) In this question, we get an equation
If we consider formula:
We can use this relation also to solve the equation at last.
(iii) Here, students should take care while squaring and adding the two equations that there should not be any calculation mistakes, otherwise the whole question will be wrong.
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




