
The minimum value of
(A).
(B).
(C).
(D).

Answer
483.3k+ views
Hint: According to the second law of motion, an external force is required to change the state of rest or motion of a body. Resolve all the forces acting on the block into its components individually for x components and y components of the forces and use the equations to find the minimum value of force.
Formulas Used:
Complete answer:
A block of mass
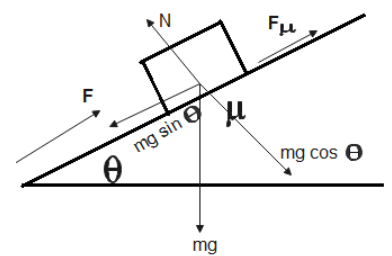
In the x-direction, the forces acting are-
Here,
Forces acting in the y-direction,
Here,
We know that,
Therefore, from eq (2), we get,
Substituting eq (3) in eq (1), we get,
Therefore, the value of
So, the correct option is (A).
Note:
Normal force is also called contact force. It is exerted by surfaces on each other to prevent them from passing through them. Frictional force is the force that opposes the motion of an object. It occurs due to the locking of irregularities between surfaces. All forces acting on the block are considered internal forces of the system as the system is at rest.
Formulas Used:
Complete answer:
A block of mass

In the x-direction, the forces acting are-
Here,
Forces acting in the y-direction,
Here,
We know that,
Therefore, from eq (2), we get,
Substituting eq (3) in eq (1), we get,
Therefore, the value of
So, the correct option is (A).
Note:
Normal force is also called contact force. It is exerted by surfaces on each other to prevent them from passing through them. Frictional force is the force that opposes the motion of an object. It occurs due to the locking of irregularities between surfaces. All forces acting on the block are considered internal forces of the system as the system is at rest.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




