
The mirror image of the curve
A.
B.
C.
D.
Answer
488.4k+ views
Hint: First of all find the locus represented by the curve. And then we will find the mirror image along the given line. We know that a complex number is
Complete step-by-step answer:
Given that,
So write the curve given above in complex number form as
Taking i common in numerator, and writing real and imaginary part as in complex number
Now to simplify the terms we will multiply numerator and denominator by
Now multiply the terms in numerator and denominator will be
Since
Multiplying the terms in numerators and separating the real and imaginary parts
The terms xy cancels here and we get
Now here we separated the real and imaginary terms.
Given that
In this case real and imaginary parts are the same. So equating them,
Cancelling the denominators from both the sides
Adding x and y terms,
Now if we observe this is the locus of a circle with center
It can be plotted as ,
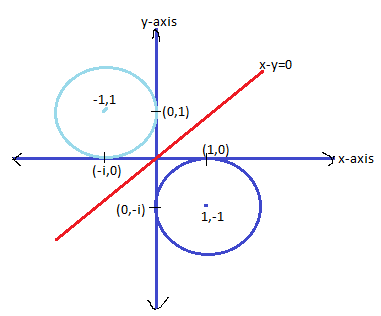
Now since the locus of light blue circle is the mirror image of the curve. Thus the curve is given as
Hence option C is the correct answer.
Note: Students generally make mistakes when we simplify the fraction with the help of complex conjugate of term in denominator. So take the simplification term carefully. And the mirror image of the curve is along the line x=y so draw that line to get an exact idea of the mirror locus.
Complete step-by-step answer:
Given that,
So write the curve given above in complex number form as
Taking i common in numerator, and writing real and imaginary part as in complex number
Now to simplify the terms we will multiply numerator and denominator by
Now multiply the terms in numerator and denominator will be
Since
Multiplying the terms in numerators and separating the real and imaginary parts
The terms xy cancels here and we get
Now here we separated the real and imaginary terms.
Given that
In this case real and imaginary parts are the same. So equating them,
Cancelling the denominators from both the sides
Adding x and y terms,
Now if we observe this is the locus of a circle with center
It can be plotted as ,

Now since the locus of light blue circle is the mirror image of the curve. Thus the curve is given as
Hence option C is the correct answer.
Note: Students generally make mistakes when we simplify the fraction with the help of complex conjugate of term in denominator. So take the simplification term carefully. And the mirror image of the curve is along the line x=y so draw that line to get an exact idea of the mirror locus.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




