
The moment of inertia of a rod of mass M and length L about an axis passing through on edge of perpendicular to its length will be:
A.
B.
C.
D.
Answer
513.9k+ views
Hint – In this question we need to know the moment of inertia about an axis perpendicular to the rod and then apply parallel and perpendicular theorem, to find the required moment of inertia about the given axis.
Formula used – 1)
1) Parallel theorem:
Complete step-by-step answer:
Inertia is the measure of resistance that a body of a certain mass offers when plunged into motion or, on the contrary, bought to a halt by an external force. Inertia, or the tendency of objects to resist change, varies with mass. Heavier objects are difficult to accelerate when at rest and equally difficult to stop when in motion, as compared to lighter objects.
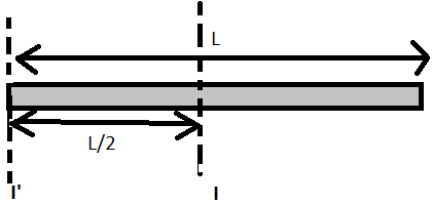
Given,
Length =L
Mass = M
We know that the moment of inertia about an axis perpendicular to the rod and passing through its centre is
Applying parallel theorem, we get
Hence, the correct option is C.
Note – In this type of question we need to remember their M.O.I. about an axis perpendicular to the object and then we can easily apply parallel and perpendicular theorems to get the desired conditions of the axis. Rotational inertia is significant in almost all physical problems involving mass in rotational motion. It is used to measure angular momentum which helps one to understand (by retaining angular momentum) how rotational motion varies as the distribution of mass changes. Inertia is directly proportional to the body's density. If you're going to feel inertia, then I'd say you drive a car and a paper ball. The car has more mass, and thus more inertia.
Formula used – 1)
1) Parallel theorem:
Complete step-by-step answer:
Inertia is the measure of resistance that a body of a certain mass offers when plunged into motion or, on the contrary, bought to a halt by an external force. Inertia, or the tendency of objects to resist change, varies with mass. Heavier objects are difficult to accelerate when at rest and equally difficult to stop when in motion, as compared to lighter objects.

Given,
Length =L
Mass = M
We know that the moment of inertia about an axis perpendicular to the rod and passing through its centre is
Applying parallel theorem, we get
Hence, the correct option is C.
Note – In this type of question we need to remember their M.O.I. about an axis perpendicular to the object and then we can easily apply parallel and perpendicular theorems to get the desired conditions of the axis. Rotational inertia is significant in almost all physical problems involving mass in rotational motion. It is used to measure angular momentum which helps one to understand (by retaining angular momentum) how rotational motion varies as the distribution of mass changes. Inertia is directly proportional to the body's density. If you're going to feel inertia, then I'd say you drive a car and a paper ball. The car has more mass, and thus more inertia.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




