
The moment of inertia of a square lamina about the perpendicular axis through its centre of mass is
A).
B).
C).
D).
Answer
440.2k+ views
3 likes
Hint: For solving this problem, we need to know the formula for Moment of Inertia of a square lamina whose axis is passing through the centre and perpendicular to it (its value is given), along with the theorem of parallel axis and perpendicular axis to find the answer.
Formula used: The moment of Inertia of a square lamina whose axis is passing through the centre and perpendicular to it is given by:
Here L is known as the length of the square lamina and M is its mass.
The theorem of parallel axis is given as:
Theorem of perpendicular axis is:
Complete step by step answer:
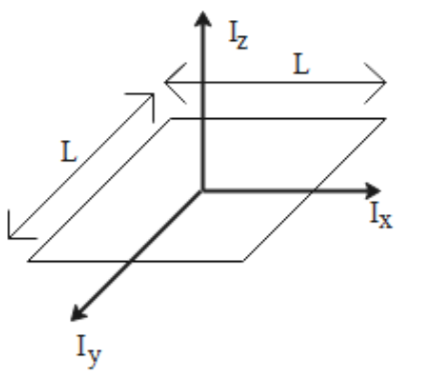
We are given the moment of inertia of a square lamina about the perpendicular axis through its centre of mass to be
Here
From this expression, we get that
Now we will use the perpendicular axis theorem. Since the lamina has a shape of square, we have
Now, using the parallel axis theorem we will find the axis parallel to
This is the required answer. Hence the correct answer is option C.
Note: In the parallel axis theorem the moment of inertia of the axis perpendicular to the centre of mass should be known in order to find the Moment of inertia for a parallel axis which is ‘h’ distance apart or vice versa. Means of the two parallel axis one should be passing through the Centre of mass of the rotating body.
Formula used: The moment of Inertia of a square lamina whose axis is passing through the centre and perpendicular to it is given by:
Here L is known as the length of the square lamina and M is its mass.
The theorem of parallel axis is given as:
Theorem of perpendicular axis is:
Complete step by step answer:

We are given the moment of inertia of a square lamina about the perpendicular axis through its centre of mass to be
Here
From this expression, we get that
Now we will use the perpendicular axis theorem. Since the lamina has a shape of square, we have
Now, using the parallel axis theorem we will find the axis parallel to
This is the required answer. Hence the correct answer is option C.
Note: In the parallel axis theorem the moment of inertia of the axis perpendicular to the centre of mass should be known in order to find the Moment of inertia for a parallel axis which is ‘h’ distance apart or vice versa. Means of the two parallel axis one should be passing through the Centre of mass of the rotating body.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
EMI starts from ₹3,108.34 per month
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




