
The moment of inertia of a uniform circular disc of radius
A.)
B.)
C.)
D.)
Answer
494.4k+ views
Hint: The moment of inertia of a uniform circular disc about an axis passing through the edge of the disc and normal to the surface can be calculated using the parallel axis theorem and form the knowledge of the moment of inertia of the circular disc about an axis passing through its centre and perpendicular to the surface.
Formula used:
The parallel axis theorem states that the moment of inertia
where
Complete step by step answer:
We can find out the moment of inertia of the uniform circular disc about an axis passing through its edge and normal to its surface by using the parallel axis theorem and the moment of inertia of the disc about the axis passing through its centre and normal to its surface.
The parallel axis theorem states that the moment of inertia
where
Therefore, let us analyze the question.
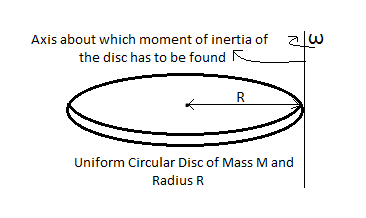
The mass of the disc is
The perpendicular distance between the edge of the disc and the centre will be nothing but the radius of the disc.
The moment of inertia of the disc about the axis passing through its centre and normal to its surface is
Therefore, using this information in (1), we get the moment of inertia
Hence, the required moment of inertia about the required axis is
Therefore, the correct option is C)
Note: Students must properly understand the parallel and perpendicular axis theorems as they are very important in solving almost all problems of rotational mechanics. This is because even if the student knows the moment of inertia about only one axis of a shape, they can easily find out the moment of inertia about other axes also using a combination of the parallel and perpendicular axis theorems.
Students must take care of the axis about which the moment of inertia is required. Usually, the moment of inertia of a body is different about different axes and hence, assuming the moment of inertia about a wrong axis will lead to a wrong value of the moment of inertia and eventually lead to a wrong answer.
ω
Formula used:
The parallel axis theorem states that the moment of inertia
where
Complete step by step answer:
We can find out the moment of inertia of the uniform circular disc about an axis passing through its edge and normal to its surface by using the parallel axis theorem and the moment of inertia of the disc about the axis passing through its centre and normal to its surface.
The parallel axis theorem states that the moment of inertia
where
Therefore, let us analyze the question.

The mass of the disc is
The perpendicular distance between the edge of the disc and the centre will be nothing but the radius of the disc.
The moment of inertia of the disc about the axis passing through its centre and normal to its surface is
Therefore, using this information in (1), we get the moment of inertia
Hence, the required moment of inertia about the required axis is
Therefore, the correct option is C)
Note: Students must properly understand the parallel and perpendicular axis theorems as they are very important in solving almost all problems of rotational mechanics. This is because even if the student knows the moment of inertia about only one axis of a shape, they can easily find out the moment of inertia about other axes also using a combination of the parallel and perpendicular axis theorems.
Students must take care of the axis about which the moment of inertia is required. Usually, the moment of inertia of a body is different about different axes and hence, assuming the moment of inertia about a wrong axis will lead to a wrong value of the moment of inertia and eventually lead to a wrong answer.
ω
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




