
The moment of inertia of a uniform semi circular disc about the axis passing through the centre of mass and perpendicular to its plane is (Mass of the disc is M and radius is R)
Answer
444.2k+ views
1 likes
Hint: In the above question we are asked to determine the moment of inertia along the axis perpendicular to the plane through the centre of mass. First we will determine the moment of inertia along the centre of the semicircular disc. Further using the parallel axis theorem of moment of inertia we will determine the moment of inertia along the centre of mass.
Formula used:
Complete step-by-step answer:
To begin with let us discuss the moment of inertia along the centre of the circle. Let us say the mass of a circular disc is 2M and the radius of the disc be R. Hence the moment of inertia of the circular disc about its centre is ,
Since the above semicircular disc has a uniform symmetric distribution of mass M, therefore we can say that the moment of inertia of the semicircular disc about its centre will be half of the moment of inertia of the circular disc i.e.
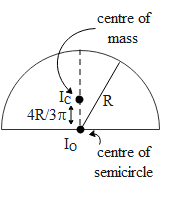
In the above figure we can see that the centre of mass is at a distance of
We know that moment of inertia along the centre of the semicircle is
Hence the correct answer of the above question is option b.
So, the correct answer is “Option b”.
Note: It is to be noted that the above semicircular disc is given to be symmetric. Hence we could say the moment of inertia of the semicircular disc is half to that of the circular disc of the same radius. If we observe the above equation of moment of inertia along the centre of mass, we can conclude that the moment of inertia about the centre of mass is the minimum.
Formula used:
Complete step-by-step answer:
To begin with let us discuss the moment of inertia along the centre of the circle. Let us say the mass of a circular disc is 2M and the radius of the disc be R. Hence the moment of inertia of the circular disc about its centre is ,
Since the above semicircular disc has a uniform symmetric distribution of mass M, therefore we can say that the moment of inertia of the semicircular disc about its centre will be half of the moment of inertia of the circular disc i.e.

In the above figure we can see that the centre of mass is at a distance of
We know that moment of inertia along the centre of the semicircle is
Hence the correct answer of the above question is option b.
So, the correct answer is “Option b”.
Note: It is to be noted that the above semicircular disc is given to be symmetric. Hence we could say the moment of inertia of the semicircular disc is half to that of the circular disc of the same radius. If we observe the above equation of moment of inertia along the centre of mass, we can conclude that the moment of inertia about the centre of mass is the minimum.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




