Question

The moment of inertia of triangular lamina of mass M and height H about its base is

$\begin{align}
  & \text{A}\text{. }\dfrac{1}{2}M{{H}^{2}} \\
 & \text{B}\text{. }\dfrac{1}{3}M{{H}^{2}} \\
 & \text{C}\text{. }\dfrac{1}{6}M{{H}^{2}} \\
 & \text{D}\text{. }\dfrac{2}{3}M{{H}^{2}} \\
\end{align}$

Answer 1

Hint: To find the moment of inertia of a triangular lamina first differentiate it into rectangular stripes and find the moment of inertia of one such stripe after that if you integrate it by taking limits applicable for the whole lamina, you will get moment of inertia of entire lamina.

Complete step by step answer:
To find the moment of inertia of triangular lamina about its base:
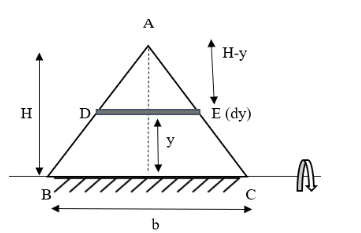
Consider a triangular lamina of base (b), altitude (h) and mass (M) as shown below

Thus, the moment of inertia of a distributed mass system can be written as
$I=\int{{{y}^{2}}}dm$ ….. (1)
Where, I is the moment of inertia, dm is the mass of a small element considered on the object, and y is the distance of the elemental mass from the axis.
Now let us differentiate this triangular lamina into rectangular stripes and consider one such stripe DE of width ‘dy’ at a distance’ y’ from the axis of rotation.
Now to find dm we have,
$dm=\dfrac{M}{A}dA$ …….. equation (2)
 M - is the total mass of triangular lamina
 A – is the area of the total triangular lamina and is given by,
A= $\dfrac{1}{2}$×base× height
$\therefore A=\dfrac{1}{2}\times b\times H$
dA - is the area of the considered stripe. To find it, consider two similar triangles ∆ADE and ∆ABC we get,
$\begin{align}
  & \dfrac{DE}{BC}=\dfrac{H-y}{H} \\
 & \\
 & DE=\dfrac{(H-y)b}{H} \\
\end{align}$
Where $BC=b$
Now area of stripe,$DE=dA=\dfrac{(H-y)b}{H}dy$
On substituting these values in (2). We get,
$\begin{align}
  & dm=\dfrac{M}{\dfrac{1}{2}\times b\times H}\dfrac{(H-y)b}{H}dy \\
 & dm=\dfrac{2M(H-y)b}{b{{H}^{2}}}dy \\
\end{align}$
Now, we got dm substitute this in (1), we get
$I=\int{\dfrac{2M(H-y)b{{y}^{2}}}{b{{H}^{2}}}}dy$
By taking constants outside, cancelling the common ‘b’ and multiplying ${{y}^{2}}$ inside we get
$I=\dfrac{2M}{{{H}^{2}}}\int_{0}^{H}{(H{{y}^{2}}}-{{y}^{3}})dy$
Limits are taken from 0to height H to cover the entire lamina. On integrating,
$I=\dfrac{2M}{{{H}^{2}}}\mathop{\left[ \dfrac{H{{y}^{3}}}{3}-\dfrac{{{y}^{4}}}{4} \right]}_{0}^{H}$
substitute the limits then and cancelling the common terms,
$\begin{align}
  & I=\dfrac{2M}{{{H}^{2}}}\left[ \dfrac{{{H}^{4}}}{3}-\dfrac{{{H}^{4}}}{4} \right] \\
 & \\
 & I=\dfrac{2M{{H}^{4}}}{{{H}^{2}}}\left[ \dfrac{1}{3}-\dfrac{1}{4} \right] \\
 & \\
 & I=2M{{H}^{2}}\left[ \dfrac{4-3}{12} \right] \\
 & \\
 & I=\dfrac{M{{H}^{2}}}{6} \\
\end{align}$
Thus, a moment of inertia of triangular lamina about its base H is found. Correct option is C.

Note: Another method for calculating mass element ‘dm’ is by using below formula and methods:
First finding surface mass density which is nothing but total mass per unit area and then multiplying it by total area.
i.e., $\sigma =\dfrac{M}{A}$
where, σ is mass density
 M and A are total mass and total area of the body, respectively.
Then, $dm=\sigma dA$.