
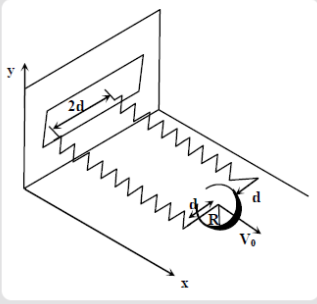
The net external force acting on the disk when its centre of mass is at displacement x with respect to its equilibrium position is

Answer
505.5k+ views
Hint:
Net Force acting on a body is given by the formula:
Where,
m is the mass of the body
Moment of Force is called Torque.
Net Torque acting on a body is given by the formula,
Where,
I is the moment of inertia of the body
Also,
Moment of inertia of a disk is given by the formula,
Using all the above formulas, we can easily compute the result.
Complete step by step solution: Net Force acting on a body is given by the formula:
Where,
m is the mass of the body
We will insert
Where,
k is the spring constant
x is the distance by which the spring has been stretched
F is the friction force on the disk
M is the mass of disk
Net Torque acting on a body is given by the formula,
Where,
I is the moment of inertia of the body
Additionally, Torque is also calculated as follows,
Where,
R is the distance of the Force from the centre of mass of the body
Now combining equations 2 and 3,
We get,
In Pure Rolling condition,
Moment of inertia of a disk is given by the formula,
Inserting the values of
We get,
Inserting the value of F in equation 1,
We get,
Hence, Option (D) is correct.
Note:
We have used a negative sign because the disk has been displaced away from the equilibrium position. Hence this force will tend to bring the disk back to its initial position.
Where,
m is the mass of the body
Moment of Force is called Torque.
Net Torque acting on a body is given by the formula,
Where,
I is the moment of inertia of the body
Also,
Moment of inertia of a disk is given by the formula,
Using all the above formulas, we can easily compute the result.
Complete step by step solution: Net Force acting on a body is given by the formula:
Where,
m is the mass of the body
We will insert
Where,
k is the spring constant
x is the distance by which the spring has been stretched
F is the friction force on the disk
M is the mass of disk
Net Torque acting on a body is given by the formula,
Where,
I is the moment of inertia of the body
Additionally, Torque is also calculated as follows,
Where,
R is the distance of the Force from the centre of mass of the body
Now combining equations 2 and 3,
We get,
In Pure Rolling condition,
Moment of inertia of a disk is given by the formula,
Inserting the values of
We get,
Inserting the value of F in equation 1,
We get,
Hence, Option (D) is correct.
Note:
We have used a negative sign because the disk has been displaced away from the equilibrium position. Hence this force will tend to bring the disk back to its initial position.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




