
The number of enantiomers of camphor is
A. four
B. three
C. two
D. one

Answer
473.7k+ views
1 likes
Hint:Enantiomers are optical active compounds. The presence of a chiral centre is necessary for a compound to be optically active. The maximum valency shown by carbon is four. A carbon having four different substituents is known as a chiral centre. We will determine the chiral centre then we will use the formula to calculate the number of enantiomers.
Complete solution:
The compounds that are mirror images of each other and are non-superimposable are known as enantiomers. Both enantiomers of an enantiomeric pair rotates the light in opposite directions. The enantiomer that rotates the light in a clockwise direction are known as dextrorotatory and represented by
A carbon attached with four different types of groups is known as a chiral centre.
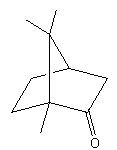
The given structure of the camphor is and chiral centre are shown as follows:

Carbon-one and carbon-fourth both are chiral centres as both carbon atoms have four different substituents.
So, the number of chiral centres in camphor is
The formula to determine the number of enantiomers is as follows:
No. of enantiomers =
Where,
n is the number of chiral centres.
On substituting
So, the number of enantiomers of camphor is
The enantiomers of camphor are shown as follows:

Both above structures are mirror images of each other and are non-superimposable.
Therefore, option (C) two, is correct.
Note:The compounds that are not mirror images of each other and have different configurations are known as diastereomers. The total number of stereoisomers is calculated by the formula
Complete solution:
The compounds that are mirror images of each other and are non-superimposable are known as enantiomers. Both enantiomers of an enantiomeric pair rotates the light in opposite directions. The enantiomer that rotates the light in a clockwise direction are known as dextrorotatory and represented by
A carbon attached with four different types of groups is known as a chiral centre.
The given structure of the camphor is and chiral centre are shown as follows:

Carbon-one and carbon-fourth both are chiral centres as both carbon atoms have four different substituents.
So, the number of chiral centres in camphor is
The formula to determine the number of enantiomers is as follows:
No. of enantiomers =
Where,
n is the number of chiral centres.
On substituting
So, the number of enantiomers of camphor is
The enantiomers of camphor are shown as follows:

Both above structures are mirror images of each other and are non-superimposable.
Therefore, option (C) two, is correct.
Note:The compounds that are not mirror images of each other and have different configurations are known as diastereomers. The total number of stereoisomers is calculated by the formula
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




