
The opposite angles of a parallelogram are (3x-2) and (x+48). Find the measure of each angle of the parallelogram.
Answer
505.2k+ views
1 likes
Hint: We know the property of a parallelogram that opposite angles of a parallelogram are equal to each other. And it is given that opposite angles of the parallelogram are (3x-2) and (x+48). We also know the property that the sum of adjacent angles of a parallelogram is equal to
Complete step-by-step answer:
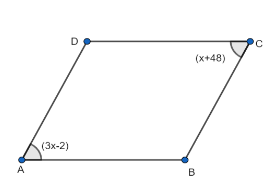
We have a parallelogram ABCD whose opposite angles are (3x-2) and (x+48).
We know the property of a parallelogram that the measure of opposite angles is equal to each other.
Here,
According to the question, it is given that the opposite angles are (3x-2) and (x+48).
Let us assume,
From equation (1), equation (2), and equation (3), we get
Now, putting the value of x in equation (2) and equation (3), we get
We also know the property of a parallelogram that the sum of adjacent angles of a parallelogram is equal to
Here, the angle
From equation (4) and equation (5), we get
We have the property that in a parallelogram the measure of opposite angles is equal to each other.
Here,
From equation (6) and equation (7), we have
From equation (4), equation (5), and equation (8), we have
Hence, the angles are
Note: In this question, one might do a mistake in the property. One can think that the sum of the opposite angles of a parallelogram is equal to
Complete step-by-step answer:

We have a parallelogram ABCD whose opposite angles are (3x-2) and (x+48).
We know the property of a parallelogram that the measure of opposite angles is equal to each other.
Here,
According to the question, it is given that the opposite angles are (3x-2) and (x+48).
Let us assume,
From equation (1), equation (2), and equation (3), we get
Now, putting the value of x in equation (2) and equation (3), we get
We also know the property of a parallelogram that the sum of adjacent angles of a parallelogram is equal to
Here, the angle
From equation (4) and equation (5), we get
We have the property that in a parallelogram the measure of opposite angles is equal to each other.
Here,
From equation (6) and equation (7), we have
From equation (4), equation (5), and equation (8), we have
Hence, the angles are
Note: In this question, one might do a mistake in the property. One can think that the sum of the opposite angles of a parallelogram is equal to
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE




