Question

The outer and inner diameters of a circular pipe are 6 cm and 4 cm respectively. If its length is 10 cm then what is the total surface area in square centimeters?
A. \[55\pi \]
B. \[110\pi \]
C. \[150\pi \]
D. None of the above

Answer 1

Hint: Here we need to find the total surface area of a pipe. We know that the pipe is in the shape of a hollow cylinder. So we will use the formula of area of a hollow cylinder to calculate the area of a pipe. We will substitute the value of the outer radius, inner radius and the length in the formula of the hollow cylinder to get the find value of the total surface area of the pipe.

Complete step by step solution:
Here we need to find the total surface area of a pipe. The given pipe is in the shape of a hollow cylinder.
It is given that the outer diameter of a pipe is 6 cm and the inner diameter of a circular pipe is 4 cm.
Now, we will draw the figure using the given information.

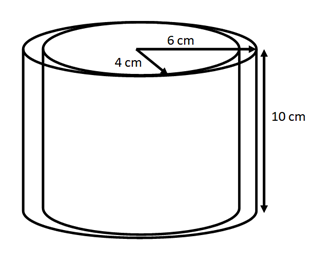
We know that the formula for the area of a hollow cylinder is given by
$Area = 2\pi h\left( {{r_2} + {r_1}} \right) + 2\pi \left( {{r_2}^2 - {r_1}^2} \right)$
${r_2} = \dfrac{6}{2}cm = 3cm$ , ${r_1} = \dfrac{4}{2}cm = 2cm$ and $h = 10cm$
Now, we will substitute the value of outer radius, inner radius and the length of a pipe in the formula.
$ \Rightarrow Area = 2 \cdot \pi \cdot 10 \cdot \left( {3 + 2} \right) + 2 \cdot \pi \cdot \left( {{3^2} - {2^2}} \right)$
On simplifying the terms, we get
$ \Rightarrow Area = 2 \cdot \pi \cdot 10 \cdot 5 + 2 \cdot \pi \cdot 5$
On multiplying the terms, we get
$ \Rightarrow Area = 100\pi + 10\pi $
On adding the terms, we get
$ \Rightarrow Area = 110\pi c{m^2}$

Hence, the total surface area of a pipe is equal to $110\pi c{m^2}$.
Hence, the correct answer is option B.

Note:
Remember that the total surface area of a hollow cylinder is equal to lateral surface area and the area of the solid bases. To find the total surface area of a hollow cylinder, we need both outer and inner radii of the hollow cylinder and the length of the hollow cylinder.