
The packing efficiency of the face centred cubic (fcc), body-centred cubic (bcc) and simple primitive cubic (pc) lattices follows the order:
A. fcc > bcc > pc
B. bcc > fcc > pc
C. pc > bcc > fcc
D. bcc > pc > fcc
Answer
516.9k+ views
Hint: We know that each cube has 8 corners, 12 edges, 6 faces, 12 face diagonals and 8 body diagonals. Keeping this information in mind we need to proceed for the comparison.
Step by step answer:
The percentage efficiency of a simple cubic unit cell is:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
Total volume of the unit cell
Number of atoms per unit cell
Volume of the atom
Thus, the percentage of occupied volume or packing efficiency
The percentage efficiency of a body-centred unit cell is:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
In this unit cell,
Total volume of the unit cell
Number of atoms per unit cell
Volume of two atoms
Therefore, packing fraction (3D)
Thus, the percentage of occupied volume or packing efficiency
The percentage efficiency of a face-centred cubic unit cell:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
In this unit cell,
Total volume of the unit cell
Number of atoms per unit cell
Volume of four atoms
(This is the occupied volume)
Therefore, packing fraction (3D)
Thus, the percentage of occupied volume or packing efficiency
Hence we can see that the packing efficiency goes in to order:
fcc > bcc > pc
So Option A is the correct answer.
Note:
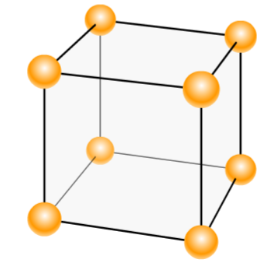
Atomic radius of simple cubic unit cell is:
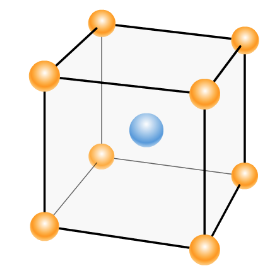
Atomic radius of body-centred unit cell is:
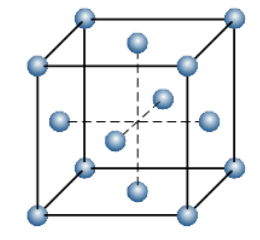
Atomic radius of face-centred unit cell is:
Step by step answer:
The percentage efficiency of a simple cubic unit cell is:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
Total volume of the unit cell
Number of atoms per unit cell
Volume of the atom
Thus, the percentage of occupied volume or packing efficiency
The percentage efficiency of a body-centred unit cell is:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
In this unit cell,
Total volume of the unit cell
Number of atoms per unit cell
Volume of two atoms
Therefore, packing fraction (3D)
Thus, the percentage of occupied volume or packing efficiency
The percentage efficiency of a face-centred cubic unit cell:
Suppose,
Length of the unit cell
Radius of the sphere (atom)
In this unit cell,
Total volume of the unit cell
Number of atoms per unit cell
Volume of four atoms
(This is the occupied volume)
Therefore, packing fraction (3D)
Thus, the percentage of occupied volume or packing efficiency
Hence we can see that the packing efficiency goes in to order:
fcc > bcc > pc
So Option A is the correct answer.
Note:

Atomic radius of simple cubic unit cell is:

Atomic radius of body-centred unit cell is:

Atomic radius of face-centred unit cell is:
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




