
The parabola
Answer
491.7k+ views
1 likes
Hint: To solve this question, we will start with finding the value of p, then on putting the value of points
Complete step-by-step answer:
We have been given a parabola
Since, parabola
Now on putting
So, we get,
Now on comparing above equation with the standard form of parabola,
So, we get
On drawing graph of the equation,
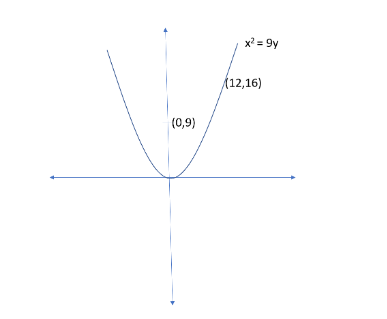
Now, from the figure we get that we need to find the distance from
We will find the focal distance using distance formula, which is
Distance formula
Let the distance be q, so using distance formula mentioned above, we get
So, the focal distance of the point is
Note: In the question, we are asked about the focal distance of the point. Focal length is the distance between the vertex and focus, it is measured along the focus of symmetry. That’s why in the question we have found the distance between
Complete step-by-step answer:
We have been given a parabola
Since, parabola
Now on putting
So, we get,
Now on comparing above equation with the standard form of parabola,
So, we get
On drawing graph of the equation,

Now, from the figure we get that we need to find the distance from
We will find the focal distance using distance formula, which is
Distance formula
Let the distance be q, so using distance formula mentioned above, we get
So, the focal distance of the point is
Note: In the question, we are asked about the focal distance of the point. Focal length is the distance between the vertex and focus, it is measured along the focus of symmetry. That’s why in the question we have found the distance between
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




