
The path is a projectile is a parabola.
A). True
B). False
Answer
511.5k+ views
Hint: Divide the initial velocity of the object into the horizontal component and vertical component. Then analyse each component separately to get the expression for the position of the object. Use the kinematic equations wherever required.
Formula used:
Complete step by step answer:
Suppose an object is projected from the ground with an initial velocity of

Divide the velocity ‘u’ into the components along the axis (x component and y component), as shown in the figure. Now we will analyse these two components separately.
Let us first analyse the x component i.e. the velocity along the positive x-axis direction. The value of the x component (ux) will be
Now, let us analyse the y component of the initial velocity (
Therefore,
Therefore, we found out how the position of the object changes with time. Now, substitute the value of t from equation (1) into equation (2)
We get,
Make the coefficient of
Since, u, g and
Therefore, we can write the equation (3) as,
Use completing the square method on the left hand side of the equation.
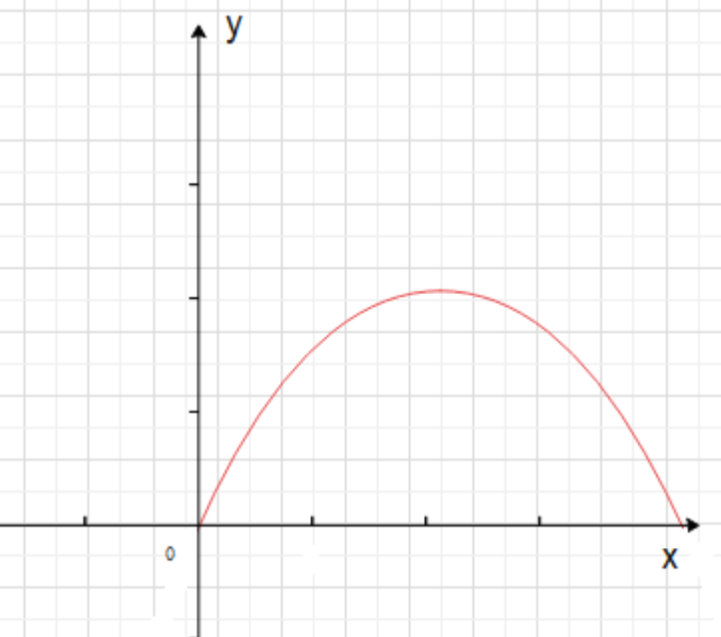
The above equation is an equation of a parabola passing through the origin with
Hence, the correct option is (A)true.
Note: We proved that the path of a projectile is a parabola. However, it is true only in ideal condition. In practical cases, this does not happen. In this solution, we have neglected the air resistance, which opposes the motion of an object. Hence, the projectile will not take a parabolic path. One more exception in this the solution is that we have taken the acceleration due to gravity (g) as constant. The value of g changes with altitude. For small heights, the change is not much and can be neglected.
Formula used:
Complete step by step answer:
Suppose an object is projected from the ground with an initial velocity of

Divide the velocity ‘u’ into the components along the axis (x component and y component), as shown in the figure. Now we will analyse these two components separately.
Let us first analyse the x component i.e. the velocity along the positive x-axis direction. The value of the x component (ux) will be
Now, let us analyse the y component of the initial velocity (
Therefore,
Therefore, we found out how the position of the object changes with time. Now, substitute the value of t from equation (1) into equation (2)
We get,
Make the coefficient of
Since, u, g and
Therefore, we can write the equation (3) as,
Use completing the square method on the left hand side of the equation.

The above equation is an equation of a parabola passing through the origin with
Hence, the correct option is (A)true.
Note: We proved that the path of a projectile is a parabola. However, it is true only in ideal condition. In practical cases, this does not happen. In this solution, we have neglected the air resistance, which opposes the motion of an object. Hence, the projectile will not take a parabolic path. One more exception in this the solution is that we have taken the acceleration due to gravity (g) as constant. The value of g changes with altitude. For small heights, the change is not much and can be neglected.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




