
The path length of one oscillation of a simple pendulum of length of
Answer
451.8k+ views
Hint :In order to solve this question, we are going to draw a schematic diagram of the pendulum and then calculate the height
The formula used in this question is
The angle
All of the potential energy at
Complete Step By Step Answer:
Let us first draw the figure for the given arrangement
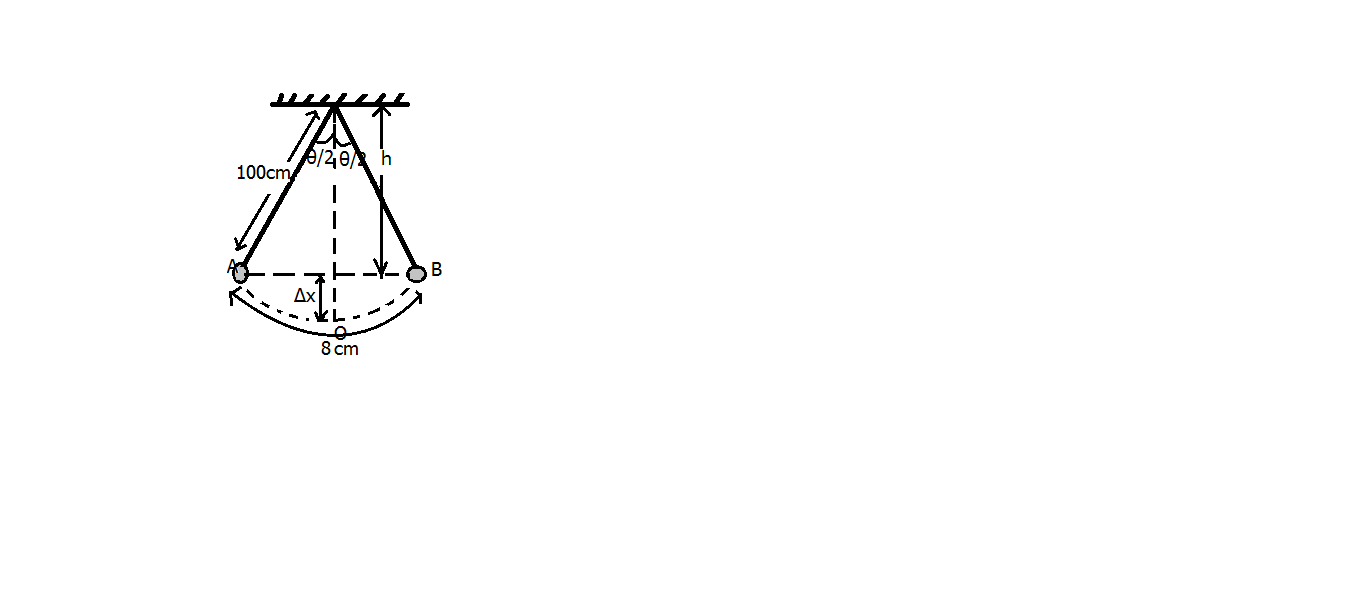
As it is given that the length of the complete oscillation is
Putting the values of the arc and the radius
So, the angles
So, the height,
Therefore,
Now, all of the potential energy at
So,
Now, as it is given that,
Since,
Therefore,
Note :
The angle
The formula used in this question is
The angle
All of the potential energy at
Complete Step By Step Answer:
Let us first draw the figure for the given arrangement

As it is given that the length of the complete oscillation is
Putting the values of the arc and the radius
So, the angles
So, the height,
Therefore,
Now, all of the potential energy at
So,
Now, as it is given that,
Since,
Therefore,
Note :
The angle
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




