
The perimeter of a kite is 80 cm. If the length of one side is 20 cm, find the length of the other side.
A. 10 cm
B. 20 cm
C. 30 cm
D. none
Answer
483k+ views
Hint: We first form the shape of the kite and try to figure out the perimeter of the kite. We assume the breadth of the kite and put that in the equation of the perimeter of the quadrilateral. We get a linear equation of x and we solve it to find the solution to the problem.
Complete step-by-step solution
The shape of a kite is of the shape of a quadrilateral. It has two long sides and two short sides and consecutive two sides as a pair are equal.
It’s given that the perimeter of a kite is 80 cm. The length of one side is 20 cm. One consecutive side is also of length 20 cm.
Let’s assume that the other two sides of the quadrilateral are x cm.
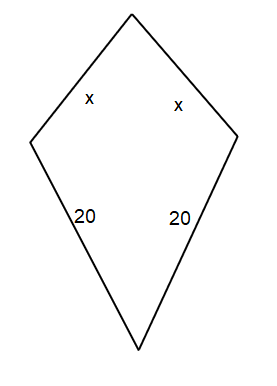
Now we will try to find the perimeter of the kite.
We know that the perimeter is the sum of the sides which is
So, the perimeter of the kite is
Now we equate both sides to find the linear equation
We solve the equation to find the value of x.
The breadth of the kite is 20 cm. The correct option is B.
Note: We can see that the length and the breadth of the kite were both of the same lengths. So, the figure was more square-shaped rather than a rectangle. But it is not certain for every case and that’s why we can’t assume that the perimeter would be a square. Square is a special form of a rectangle.
Complete step-by-step solution
The shape of a kite is of the shape of a quadrilateral. It has two long sides and two short sides and consecutive two sides as a pair are equal.
It’s given that the perimeter of a kite is 80 cm. The length of one side is 20 cm. One consecutive side is also of length 20 cm.
Let’s assume that the other two sides of the quadrilateral are x cm.

Now we will try to find the perimeter of the kite.
We know that the perimeter is the sum of the sides which is
So, the perimeter of the kite is
Now we equate both sides to find the linear equation
We solve the equation to find the value of x.
The breadth of the kite is 20 cm. The correct option is B.
Note: We can see that the length and the breadth of the kite were both of the same lengths. So, the figure was more square-shaped rather than a rectangle. But it is not certain for every case and that’s why we can’t assume that the perimeter would be a square. Square is a special form of a rectangle.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

How many ten lakhs are in one crore-class-8-maths-CBSE




