
The perimeter of a regular nonagon is 72 meters. Find the area of the polygon in square meters?
Answer
391.8k+ views
Hint: In the above question, we are given a nonagon whose perimeter is given as 72 meters. A polygon which has 9 sides is called a nonagon. The perimeter of the nonagon is given in meters and we have to find the area of the nonagon in square meters. In order to approach the solution, first we have to find the length of each side and then the distance of each edge from the centre of the nonagon. After that we can find the area of each triangle, formed inside the nonagon after joining the centre to the edges. Thus, the area of the nonagon can be obtained.
Complete step-by-step answer:
Given that, the perimeter of the nonagon is
Since a nonagon has
i.e.
Now join all the
Let the distance from the centre to the edges be
Now, the vertical angles of each isosceles triangle will be equal to
i.e.
Therefore, the other two angles will each be equal to
i.e.
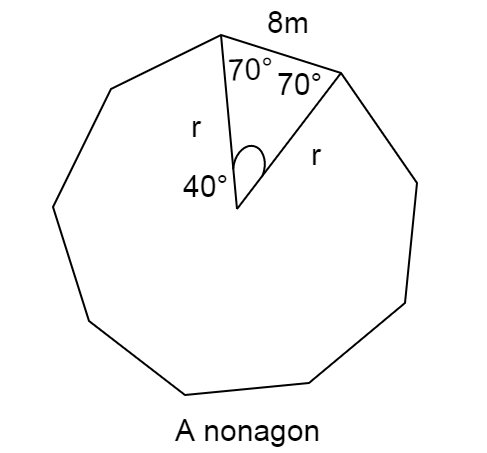
Now by Sine law, we can write
Therefore,
Now the area of each isosceles triangle can be obtained by the formula
Therefore, the total area of the nonagon is given by
Putting the value of
On solving, that gives
Or,
Putting the values of
That gives,
Or,
Hence
Therefore, the area of the nonagon is
Note: Since we obtained each side of the nonagon, now we can also obtain each interior angle of the nonagon using the formula of sum of all interior angles of a n-sided polygon which is given as,
For a nonagon,
Therefore, sum of all interior angles of a nonagon is given by
i.e.
Hence,
Therefore each angle of a nonagon is
i.e.
Therefore each angle of a nonagon is
Complete step-by-step answer:
Given that, the perimeter of the nonagon is
Since a nonagon has
i.e.
Now join all the
Let the distance from the centre to the edges be
Now, the vertical angles of each isosceles triangle will be equal to
i.e.
Therefore, the other two angles will each be equal to
i.e.

Now by Sine law, we can write
Therefore,
Now the area of each isosceles triangle can be obtained by the formula
Therefore, the total area of the nonagon is given by
Putting the value of
On solving, that gives
Or,
Putting the values of
That gives,
Or,
Hence
Therefore, the area of the nonagon is
Note: Since we obtained each side of the nonagon, now we can also obtain each interior angle of the nonagon using the formula of sum of all interior angles of a n-sided polygon which is given as,
For a nonagon,
Therefore, sum of all interior angles of a nonagon is given by
i.e.
Hence,
Therefore each angle of a nonagon is
i.e.
Therefore each angle of a nonagon is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




