
The position coordinates of a projectile from the ground on a certain planet (with no atmosphere) are given by
Answer
480.3k+ views
Hint: We are given the position coordinates of the projectile from which we can calculate the velocities of the particle along x and y-direction. Then from the projectile diagram, we can relate the angle with vertical to the velocities along the x and y-direction. Solving the obtained expression, we can find out the value of the angle.
Complete step-by-step solution:
We are given the expressions for the position coordinates of a projectile from the ground on
a certain point. The expression for the x-coordinate is given as
The expression for the y-coordinate is given as
As we know the velocity of a particle is equal to the time derivative of the position of the particle. Therefore, we can find out the velocity of the given projectile along the x and y-direction. The velocity along the x-direction can be calculated in the following way.
Similarly, the velocity along the y-direction is given as
We are asked to calculate the angle at origin and at the origin, t = 0. Therefore, we have the following values of velocities at t = 0.
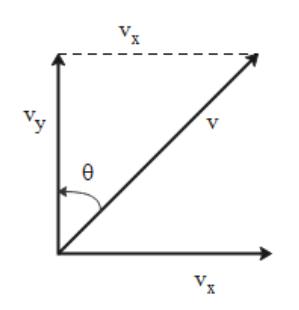
In the diagram,
This is the required value of angle. Hence, the correct answer is option B.
Note: It should be noted that the absence of atmosphere means that there is no air friction between the projectile and the medium in which it is projected. If there is some friction present then it will hinder the motion of the projectile and it will end up covering a shorter distance.
Complete step-by-step solution:
We are given the expressions for the position coordinates of a projectile from the ground on
a certain point. The expression for the x-coordinate is given as
The expression for the y-coordinate is given as
As we know the velocity of a particle is equal to the time derivative of the position of the particle. Therefore, we can find out the velocity of the given projectile along the x and y-direction. The velocity along the x-direction can be calculated in the following way.
Similarly, the velocity along the y-direction is given as
We are asked to calculate the angle at origin and at the origin, t = 0. Therefore, we have the following values of velocities at t = 0.

In the diagram,
This is the required value of angle. Hence, the correct answer is option B.
Note: It should be noted that the absence of atmosphere means that there is no air friction between the projectile and the medium in which it is projected. If there is some friction present then it will hinder the motion of the projectile and it will end up covering a shorter distance.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




