
The position time graph for motion with zero acceleration is:
A.)
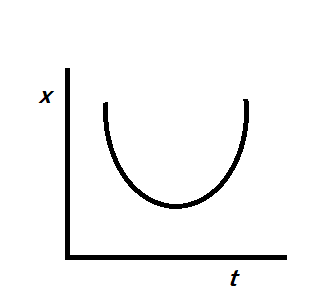
B.)
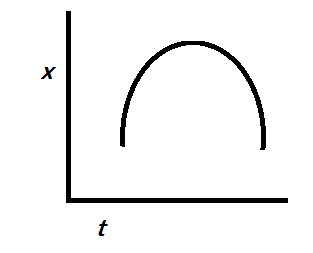
C.)
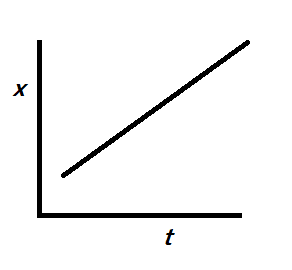
D.)
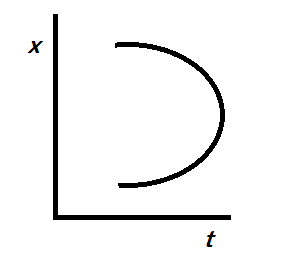
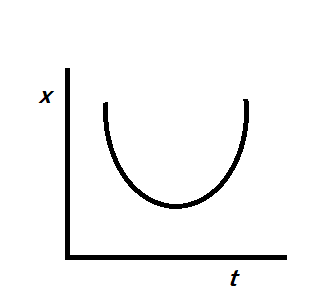
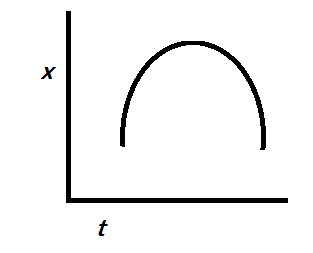
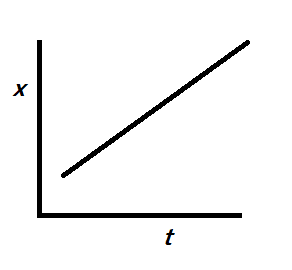
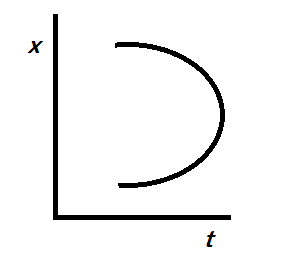
Answer
499.2k+ views
Hint: We know that acceleration is how fast or slow the velocity is with respect to time.This differentiation is nothing but the slope of the graph. Hence we must take the slope of the given curves to find the correct option, among the following.
Formula used:
Complete answer:
We know that velocity is the rate of change of displacement with respect to time. It is mathematically denoted as
Similarly, acceleration is the rate of change of velocity with respect to time. It is mathematically denoted as
Given that acceleration is zero, or
Since we know from mathematics, that
Then the v-t graph is given as:
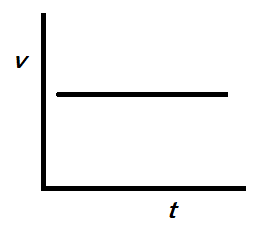
Then for
Since the x-t graph must be linear, in the given options, only C is linear and the other options are not linear i.e. specifically quadratic here.
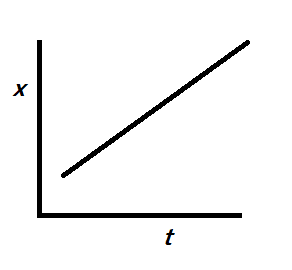
So, the correct answer is “Option C”.
Note:
Here, the answer is discussed in the form of derivation of simplicity. However one can integrate
Formula used:
Complete answer:
We know that velocity is the rate of change of displacement with respect to time. It is mathematically denoted as
Similarly, acceleration is the rate of change of velocity with respect to time. It is mathematically denoted as
Given that acceleration is zero, or
Since we know from mathematics, that
Then the v-t graph is given as:
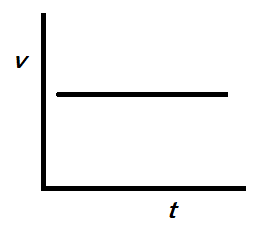
Then for
Since the x-t graph must be linear, in the given options, only C is linear and the other options are not linear i.e. specifically quadratic here.
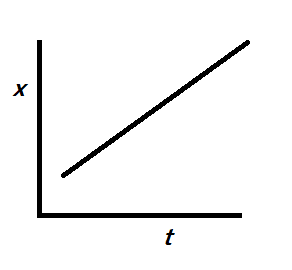
So, the correct answer is “Option C”.
Note:
Here, the answer is discussed in the form of derivation of simplicity. However one can integrate
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




