
The potential energy associated with an electron in the orbit
a) Increases with the increase in the radii of the orbit
b) Decreases with the increase in the radii of the orbit
c) Remains the same with change in the radii of the orbit
d) None of these
Answer
501.6k+ views
1 likes
Hint: The electrons revolve in the orbits with the nucleus of the atom as its centre. The nucleus has a positive charge and the electrons have a negative charge. This results in an electrostatic force of attraction between them. The electrons cannot exist in any orbit of radius r i.e. they exist in permitted orbits according to Bohr’s theory. From the definition of energy it becomes clear that whether potential energy increases or decreases with the radius due to electrostatic force.
Complete step by step answer:
The electrostatic force due a positive charge is given by
Hence the expression for potential energy is
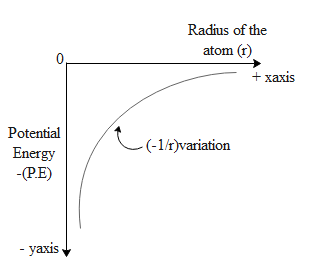
Hence, the correct answer is option A.
Note:
One may conclude that the potential energy varies or is inversely proportional to the radius of the orbit . Hence the potential energy decreases with the increase in the radius. But this is not true because the negative value of potential energy increases as we decrease the radius of the orbit.
Complete step by step answer:
The electrostatic force due a positive charge is given by
Hence the expression for potential energy is

Hence, the correct answer is option A.
Note:
One may conclude that the potential energy varies or is inversely proportional to the radius of the orbit . Hence the potential energy decreases with the increase in the radius. But this is not true because the negative value of potential energy increases as we decrease the radius of the orbit.
Latest Vedantu courses for you
Grade 10 | ICSE | SCHOOL | English
Vedantu ICSE 10 Pro Course (2025-26)
School Full course for ICSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




