
The P-T and V-T diagrams of an isochoric process of
Answer
483.3k+ views
Hint : In an isochoric process, the volume of the system remains constant while the system undergoes the process. Using the ideal gas law we can relate the pressure, volume, and temperature of an ideal gas in the initial and the final states of the gas.
Formula used: In this question, we will use the following formula:
Complete step by step answer
We’ve been given an isochoric process of
Using the ideal gas law, we can write
Since the volume of the gas remains constant, we can write
Cross multiplying the numerator and denominator, we get
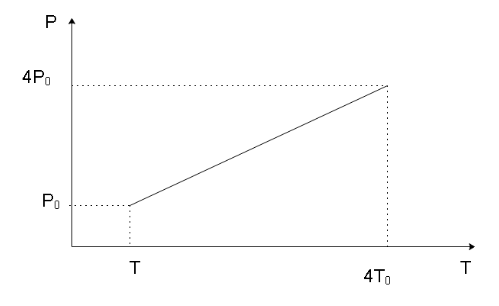
We can now form the P-T and V-T diagrams knowing the initial and final stage of the gas as shown below

Note
Since the process is isochoric, we can get a hint that in the P-V graph, the line indicating the process will be parallel to the
Formula used: In this question, we will use the following formula:
Complete step by step answer
We’ve been given an isochoric process of
Using the ideal gas law, we can write
Since the volume of the gas remains constant, we can write
Cross multiplying the numerator and denominator, we get
We can now form the P-T and V-T diagrams knowing the initial and final stage of the gas as shown below


Note
Since the process is isochoric, we can get a hint that in the P-V graph, the line indicating the process will be parallel to the
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




