Question

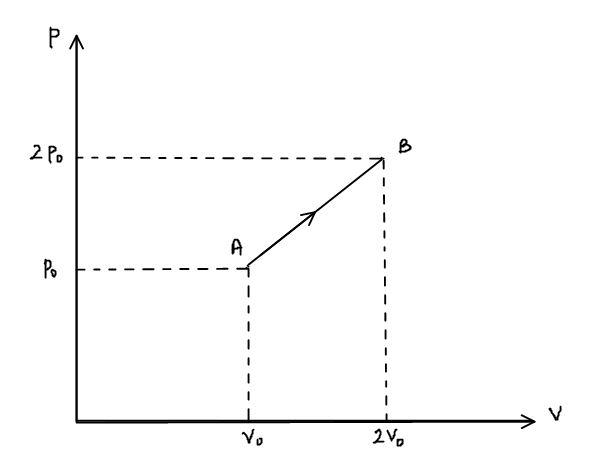
The p-v diagram of 2g of helium gas for a certain process A→B is shown in the figure below. What is the heat given to the gas during the process A→B?

A. $4{p_ \circ }{V_ \circ }$
B. $6{p_ \circ }{V_ \circ }$
C. $4.5{p_ \circ }{V_ \circ }$
D. $2{p_ \circ }{V_ \circ }$

Answer 1

Hint: In such types of questions, students should observe which type of process the graph is following and also the initial and final coordinates of the process given in the graph.

Complete Step by Step Answer:
Step 1:
As the gas is helium and the degree of freedom for helium gas is 3, i.e. $F = 3$.
Here, F is a degree of freedom.

Step 2:
Due to change in process, there will be change in internal energy of the gas, so let’s calculate the change in internal energy of the gas,
The change in internal energy of the gas is given by,
$\Delta U = \dfrac{F}{2} \cdot \Delta \left( {P \cdot V} \right)$
Here, $\Delta \left( {P \cdot V} \right)$ is \[{P_2} \cdot {V_2} - {P_1} \cdot {V_1}\] which can be calculated from the given graph.
And F is the degree of freedom and $\Delta U$ is a change in internal energy of the gas.
Substituting the value of $\Delta \left( {P \cdot V} \right)$, the relation becomes,
\[\Delta U = \dfrac{F}{2} \cdot \left( {{P_2} \cdot {V_2} - {P_1} \cdot {V_1}} \right)\]

Step 3:
The value of ${P_2} = 2{p_ \circ }$, ${V_2} = 2{V_ \circ }$, ${P_1} = {p_ \circ }$ and ${V_1} = {V_ \circ }$ substituting these values in the above relation,
We get,
\[
  \Delta U = \dfrac{3}{2} \cdot \left( {4{p_ \circ }{V_ \circ } - {p_ \circ }{V_ \circ }} \right) \\
  \Delta U = \dfrac{3}{2} \cdot \left( {3{p_ \circ }{V_ \circ }} \right) \\
  \Delta U = \dfrac{9}{2} \cdot \left( {{p_ \circ }{V_ \circ }} \right) \\
 \] ………eq.1
So, the change in internal energy is equals to \[\Delta U = \dfrac{9}{2} \cdot \left( {{p_ \circ }{V_ \circ }} \right)\].

Step 4:
The area of pressure-volume graph is equal to the work done by the gas,
Therefore, the area of trapezium is given by,
$
  W = \dfrac{1}{2} \cdot \left( {2{p_ \circ } + {p_ \circ }} \right) \cdot {V_ \circ } \\
  W = \dfrac{1}{2} \cdot 3{p_ \circ } \cdot {V_ \circ } \\
  W = \dfrac{3}{2} \cdot {p_ \circ } \cdot {V_ \circ } \\
 $………eq.2
So, the work done by the gas is given by, $W = \dfrac{3}{2} \cdot {p_ \circ } \cdot {V_ \circ }$,
Step 5:
The first law of thermodynamics says,
$\Delta Q = W + \Delta U$
Where,
$\Delta Q$ = Change in heat transfer of gas.
W = Work done by the gas.
$\Delta U$=Change in internal energy of the gas.
Putting the value of the work done by gas and change in internal energy of the gas in the above relation from equation 1 and equation 2.
We get,
\[
  \Delta Q = \dfrac{3}{2} \cdot \left( {{p_ \circ } \cdot {V_ \circ }} \right) + \dfrac{9}{2} \cdot \left( {{p_ \circ } \cdot {V_ \circ }} \right) \\
  \Delta Q = \dfrac{{12}}{2} \cdot \left( {{p_ \circ } \cdot {V_ \circ }} \right) \\
  \Delta Q = 6 \cdot \left( {{p_ \circ } \cdot {V_ \circ }} \right) \\
 \]

$\therefore$ the heat given to the gas during the process A→B is $6{p_ \circ }{V_ \circ }$. so, the correct option is (B).

Note:
- The degree of freedom of the helium is given as 3, which means translation motion is possible only in three directions.
- The area of the pressure-volume graph gives work done by the gas. Don’t get confused while taking the area of the trapezium which is formed by the process A $\to$ B.
- The area of trapezium is taken by using the formula of area of trapezium which is Area=$\dfrac{1}{2} \times $(Sum of parallel sides)$\times $(distance between the parallel sides).