
The radii of two circles are 8cms and 6cms respectively. Find the radius of the circle having an area equal to the sum of the areas of the two circles.
Answer
502.8k+ views
Hint: To solve the given question, we start by finding the area of the given circles from their given radius using the formula of area of circle is equal to
Complete step-by-step solution:
Let us consider the radius and area of the first circle as
Let us consider the radius and area of the second circle as
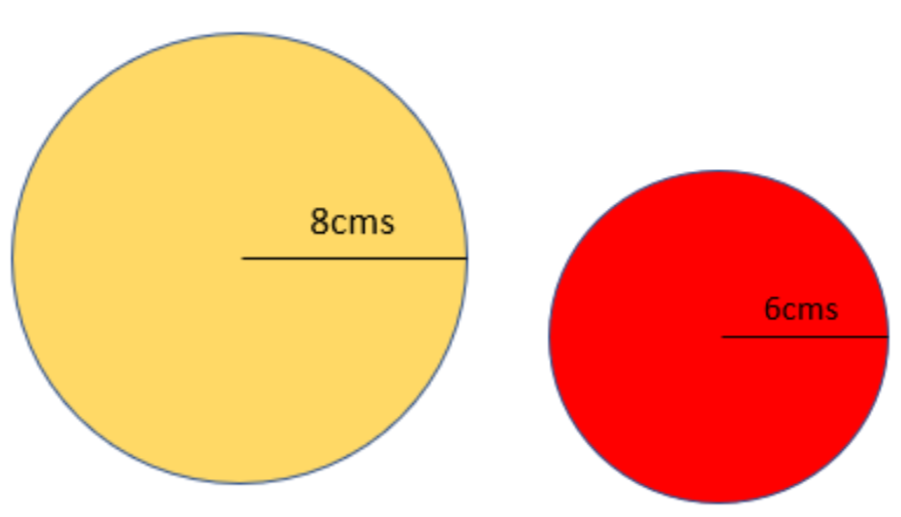
We were given that the radius of the first circle is 8cms, and the radius of the second circle is 6cms.
Given
Using the formula for Area of a circle with radius r.
So, let us use the above formula on the first circle and we get area of first circle as,
So, let us use the above formula again on the second circle and we get area of the second circle as,
Then the sum of the areas of circles is
We need to find the radius of the circle having its area equal to the sum of areas of the first and second circles.
Let them consider the radius and area of the required circle as
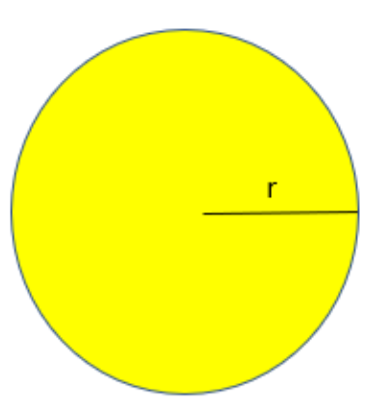
Then by using the formula of area of circle, we get
As the sum of the areas of first and second circles is equal to the area of above circle, we get
So, by using equations (3) and (4)
So, the radius of required circle is 10cms.
Hence the answer is 10cms.
Note: One can make a mistake of adding the radii of the circles to find the required radius in the given question. But as the area of the circle is proportional to the square of the radius, we should not add the radius directly to find the radius of the required circle.
Complete step-by-step solution:
Let us consider the radius and area of the first circle as
Let us consider the radius and area of the second circle as

We were given that the radius of the first circle is 8cms, and the radius of the second circle is 6cms.
Given
Using the formula for Area of a circle with radius r.
So, let us use the above formula on the first circle and we get area of first circle as,
So, let us use the above formula again on the second circle and we get area of the second circle as,
Then the sum of the areas of circles is
We need to find the radius of the circle having its area equal to the sum of areas of the first and second circles.
Let them consider the radius and area of the required circle as

Then by using the formula of area of circle, we get
As the sum of the areas of first and second circles is equal to the area of above circle, we get
So, by using equations (3) and (4)
So, the radius of required circle is 10cms.
Hence the answer is 10cms.
Note: One can make a mistake of adding the radii of the circles to find the required radius in the given question. But as the area of the circle is proportional to the square of the radius, we should not add the radius directly to find the radius of the required circle.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




