
The radius of gyration of a solid hemisphere of mass M and radius R about an axis parallel to a diameter

Answer
448.2k+ views
Hint: First we have to find the location of centre of mass of the given figure, then we have to apply theorem of parallel axis so that we can find moment of inertia also the centre of mass, then again we apply theorem of parallel axis so that we can find moment of inertia along axis AB. Finally radius of gyration formula is applied and we the required value.
Complete answer:
Since we know that the radius of gyration is the distance of the axis from the centre of mass of the body.
So centre of mass of this figure lies at the middle point on this sphere which is at distance
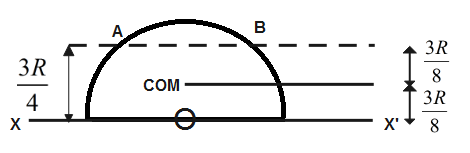
First we have to calculate the Moment of inertia of the solid sphere along the axis passing through the centre of the sphere is given by axis XX’.
Where M be the mass of the solid sphere and R be the radius of the sphere.
Now we find the moment of inertia along the axis passing through the centre of mass by applying the theorem of parallel axis.
Where h is the distance between two parallel axes.
Put the value of h=
Now we find the moment of inertia of axis AB by applying theorem of parallel axis
Where h’ is the distance between axis of centre of mass and AB axis
Put the value of h’=
Now we put the value of Moment of inertia passing through the centre of mass from equation 1,we get
Since for radius of gyration can be represented by :-
Applying this relation along the axis AB
From equation 2 and 3
So the correct option is D.
Note:
With the help of Theorem of parallel axis and theorem of perpendicular axis we can find the moment of inertia along any axis in any figure. Moment of inertia is usually specified with respect to an axis of rotation. It mainly depends also on the distribution of mass around an axis of rotation. For different distributions of mass MOI will change.
Complete answer:
Since we know that the radius of gyration is the distance of the axis from the centre of mass of the body.
So centre of mass of this figure lies at the middle point on this sphere which is at distance

First we have to calculate the Moment of inertia of the solid sphere along the axis passing through the centre of the sphere is given by axis XX’.
Where M be the mass of the solid sphere and R be the radius of the sphere.
Now we find the moment of inertia along the axis passing through the centre of mass by applying the theorem of parallel axis.
Where h is the distance between two parallel axes.
Put the value of h=
Now we find the moment of inertia of axis AB by applying theorem of parallel axis
Where h’ is the distance between axis of centre of mass and AB axis
Put the value of h’=
Now we put the value of Moment of inertia passing through the centre of mass from equation 1,we get
Since for radius of gyration can be represented by :-
Applying this relation along the axis AB
From equation 2 and 3
So the correct option is D.
Note:
With the help of Theorem of parallel axis and theorem of perpendicular axis we can find the moment of inertia along any axis in any figure. Moment of inertia is usually specified with respect to an axis of rotation. It mainly depends also on the distribution of mass around an axis of rotation. For different distributions of mass MOI will change.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




