Questions & Answers
Question
Answers
Related Questions
The radius of the circle is 15 cm and the length of one of the chords is 18cm. Find the distance of the chord from the centre.
Answer
![Verified]()
Verified
Verified
Hint: Draw a perpendicular from the center to the chord of the circle and use the property of the circle that says a perpendicular drawn from the centre of the circle to the chord bisects the chord.
Complete step-by-step answer:
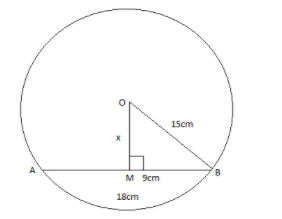
Let AB=18cm is the chord of the circle as given in the question
And OB=15cm is the radius of the circle.
Let OM be a perpendicular drawn from the centre of the circle to the chord.
Let OM=x cm
Since the perpendicular drawn on a chord from the centre of the circle bisects the chord (property of a circle)
$ \Rightarrow $ MB=9cm
Thus in the right angled triangle OMB,
Using Pythagoras theorem we get,
$O{B^2} = O{M^2} + M{B^2}$
$ \Rightarrow {(15)^2} = {x^2} + {(9)^2}$
$ \Rightarrow 225 = {x^2} + 81$
$ \Rightarrow {x^2} = 144$
$ \Rightarrow x = 12cm$
Thus the distance of the chord from the center of the circle is 12cm.
Note: It is very important in such questions to realize that we have to draw a perpendicular from the centre to the chord or else the property will not be applicable.
No right angled triangle is formed in such a case and hence is difficult to solve the question.
Complete step-by-step answer:
Let AB=18cm is the chord of the circle as given in the question
And OB=15cm is the radius of the circle.
Let OM be a perpendicular drawn from the centre of the circle to the chord.
Let OM=x cm
Since the perpendicular drawn on a chord from the centre of the circle bisects the chord (property of a circle)
$ \Rightarrow $ MB=9cm
Thus in the right angled triangle OMB,
Using Pythagoras theorem we get,
$O{B^2} = O{M^2} + M{B^2}$
$ \Rightarrow {(15)^2} = {x^2} + {(9)^2}$
$ \Rightarrow 225 = {x^2} + 81$
$ \Rightarrow {x^2} = 144$
$ \Rightarrow x = 12cm$
Thus the distance of the chord from the center of the circle is 12cm.
Note: It is very important in such questions to realize that we have to draw a perpendicular from the centre to the chord or else the property will not be applicable.
No right angled triangle is formed in such a case and hence is difficult to solve the question.
×
Sorry!, This page is not available for now to bookmark.
Like this
Liked
-
LIVECourses
-
Crash Courses 2021
-
Vedantu Pro
-
Vedantu Assist
-
Class 12
-
-
JEE
-
JEE Main & Advanced 2021
-
JEE Main & Advanced 2022
-
-
NEET
-
NEET 2021
-
NEET 2022
-
-
NDA
-
CBSE
-
Commerce
-
Class 11
-
Class 12
-
-
ICSE
-
Maharashtra Board
-
Class 9
-
Class 10
-
-
Micro Courses
-
-
FREEMaster Classes
-
PREMIUM1-on-1 Classes
-
Study Material
-
NCERT Solutions
-
Previous Year Papers
-
Mock Tests
-
Sample Papers
-
Reference Book Solutions
-
ICSE Solutions
-
School Syllabus
-
Revision Notes
-
Important Questions
-
Math Formula Sheets
-
-
More
Book your FREE Online counselling session
Share your contact information
Your FREE Online counselling session has been booked!
Vedantu academic counsellor will be calling you shortly for your Online Counselling session.
DONE
Please try again later
OK
NCERT Book Solutions
Reference Book Solutions
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
(Mon-Sun: 9am - 11pm IST)
Questions?
Chat with us
