
The range of
A.
B.
C.
D.
Answer
475.5k+ views
Hint: Here we will use the concept of trigonometric function. First, we will find the range and domain of secant function. Then with the help of this range and domain we can easily identify the range domain of the inverse secant function.
Complete step by step solution:
Let the given function
From the above equation the value of
Here we can clearly see that the
This means that the domain includes all the value from
We will draw the graph of the secant function.
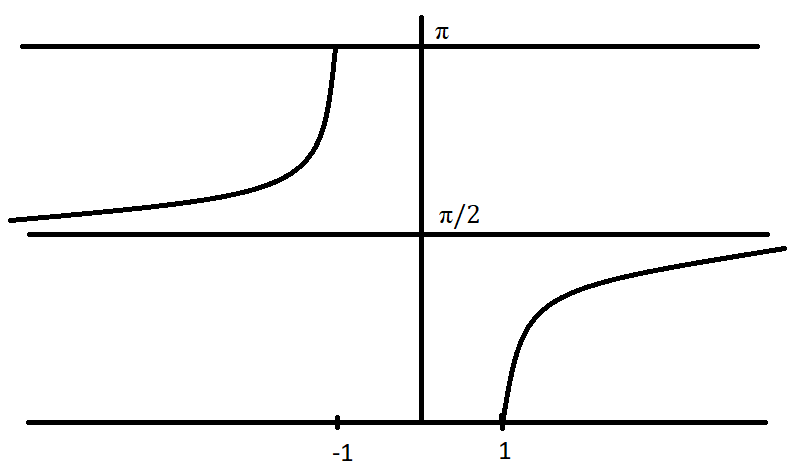
Substituting the value
Or
Hence the domain of the function
Therefore, we get range of
So, option B is the correct option.
Note: Here we should note that the range and domain of the secant function will be the range of integer value. The range and the domain of the inverse secant function will be the range of all the angles of its occurrence. The range and domain of the trigonometric functions like the value of the sin function varies from
Complete step by step solution:
Let the given function
From the above equation the value of
Here we can clearly see that the
This means that the domain includes all the value from
We will draw the graph of the secant function.

Substituting the value
Or
Hence the domain of the function
Therefore, we get range of
So, option B is the correct option.
Note: Here we should note that the range and domain of the secant function will be the range of integer value. The range and the domain of the inverse secant function will be the range of all the angles of its occurrence. The range and domain of the trigonometric functions like the value of the sin function varies from
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




