
The ratio of the area of the circumcircle and the incircle of an equilateral triangle is:
A. 4:1
B. 1:4
C. 9:1
D. 1:9
Answer
486.3k+ views
Hint: We here have been given an equilateral triangle and we have to find the ratio of the area of its circumcircle and incircle. For this, we will first mention that all the centres of an equilateral triangle lie at the same point. Then we will draw its figure and mark the both the radii of the triangle. Then we will use the property of the centroid, i.e. the centroid divides the median into a ratio of 2:1. Thus, this will be the ratio of the circumradius and the inradius. Then we will use the formula for the area of the circles which will be equal to the square of the ratio of the radii. Thus, we will get our answer.
Complete step by step answer:
We have now been given an equilateral triangle with its circumcircle and incircle and we have been asked the ratio of the areas of those circles.
Now, we know that the circumcentre and the incentre of an equilateral triangle lie at the same point.
Let us assume there to be a
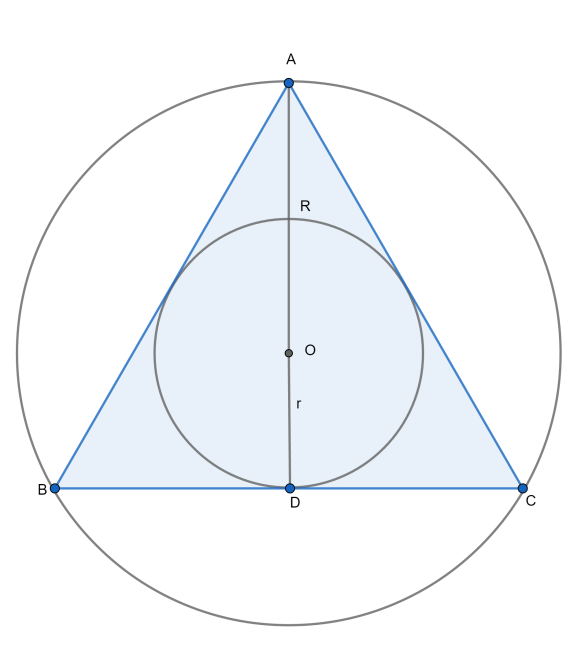
Now, we have assumed that the inradius of the triangle is ‘r’ and the circumradius is ‘R’.
We also know that in an equilateral triangle, the centroid of the triangle also lies on the same point as all the other centres. This means that the centroid, the circumcentre and the incentre lies on the same point in the triangle, i.e. O.
Now, since AD is a straight line from A to line BC passing through point O, we can say that AD is the median from A to the side BC of the
Now, from the figure, we can see that:
Now, we know that the centroid divides the median in the ratio 2:1. Thus, the point O divides the line AD in the ratio 2:1. Thus, from equation (i) we can say that:
Now, from the figure, we can see that:
Thus, we can say that:
Now, we know that the area of a circle with radius ‘a’ is given as
Thus, their ratio is given as:
Solving this, we’ll get:
Now, we know that
Thus, we get the required ratio as:
Thus the required ratio is 4:1.
So, the correct answer is “Option A”.
Note: Questions related to equilateral triangles are always simple as all the centres of the triangle lie on the same point. Not only that, every median is also an altitude, angle bisector and the perpendicular bisector of the opposite side. This is a very important property which also makes the questions related to equilateral triangles easier.
Complete step by step answer:
We have now been given an equilateral triangle with its circumcircle and incircle and we have been asked the ratio of the areas of those circles.
Now, we know that the circumcentre and the incentre of an equilateral triangle lie at the same point.
Let us assume there to be a

Now, we have assumed that the inradius of the triangle is ‘r’ and the circumradius is ‘R’.
We also know that in an equilateral triangle, the centroid of the triangle also lies on the same point as all the other centres. This means that the centroid, the circumcentre and the incentre lies on the same point in the triangle, i.e. O.
Now, since AD is a straight line from A to line BC passing through point O, we can say that AD is the median from A to the side BC of the
Now, from the figure, we can see that:
Now, we know that the centroid divides the median in the ratio 2:1. Thus, the point O divides the line AD in the ratio 2:1. Thus, from equation (i) we can say that:
Now, from the figure, we can see that:
Thus, we can say that:
Now, we know that the area of a circle with radius ‘a’ is given as
Thus, their ratio is given as:
Solving this, we’ll get:
Now, we know that
Thus, we get the required ratio as:
Thus the required ratio is 4:1.
So, the correct answer is “Option A”.
Note: Questions related to equilateral triangles are always simple as all the centres of the triangle lie on the same point. Not only that, every median is also an altitude, angle bisector and the perpendicular bisector of the opposite side. This is a very important property which also makes the questions related to equilateral triangles easier.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




