
The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
Answer
503.7k+ views
3 likes
Hint:
First, we will calculate the area of two similar triangles and then divide them. Then the similarities of two triangles are used to find the ratios of the corresponding sides.
Complete step by step solution:
Let us assume the two triangles are
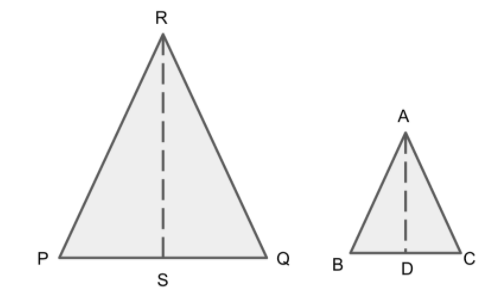
We will use the formula to find the area of triangle,
Now, we will find the area of the triangles
Dividing
Since we know that
Therefore,
Substituting this value in equation
Since we know that the triangles
Using this value in equation
Also from equation
Thus, the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
Hence, proved.
Note:
In this question, students should write the sides of the triangles appropriately. Since the general area of any triangle is
First, we will calculate the area of two similar triangles and then divide them. Then the similarities of two triangles are used to find the ratios of the corresponding sides.
Complete step by step solution:
Let us assume the two triangles are

We will use the formula to find the area of triangle,
Now, we will find the area of the triangles
Dividing
Since we know that
Therefore,
Substituting this value in equation
Since we know that the triangles
Using this value in equation
Also from equation
Thus, the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
Hence, proved.
Note:
In this question, students should write the sides of the triangles appropriately. Since the general area of any triangle is
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹42,330 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




