
The ratio of two unequal sides of a rectangle is 1:2. If the perimeter is 24 cm then the length of the diagonal is:
A)
B)
C)
D)
Answer
507k+ views
Hint: Use perimeter to find the length and breadth of the given rectangle. With the length and breadth of the rectangle, diagonal can be easily found by using Pythagoras theorem on the triangle of sides consisting of length, breadth, and diagonal.
We know that the opposite sides of the rectangle are equal. The angle between two adjacent sides is
The angle between two adjacent sides of a rectangle is
Remember that: Pythagoras theorem can be applied to every right-angled triangle.
Pythagoras theorem: square of the hypotenuse is equal to the sum of the square of base and square of perpendicular.
In a right-angled triangle, base and perpendicular are at the angle of
The comparison of two quantities in terms of ‘how many times’ is known as a ratio.
For example: There are 24 girls and 16 boys in a class. Ratio of the numbers of girls to the numbers of boys
Therefore, we can get equivalent ratios by multiplying or dividing the numerator and denominator by the same number.
A ratio can be written as a fraction, thus the ratio
Complete step by step solution:
Step 1: Draw the rectangle ABCD
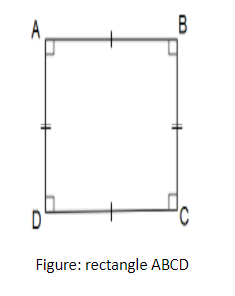
Properties of rectangle:
LENGTH: Side
BREADTH: Side
Side
All the interior angles of the rectangle ABCD
Step 2: Given that
Perimeter of rectangle
Ratio of two unequal sides of rectangle
Step 3: multiply numerator and denominator by
Step 4: find length and breadth of rectangle using parameter of rectangle
Given Parameter of rectangle = 24 cm
On substituting the corresponding values,
On simplifying expressions,
On further simplification, we get
Hence, length
And breadth
Step 5: Calculate diagonal BD using Pythagoras theorem
Join the Vertex BD, i.e. diagonal of rectangle ABCD.
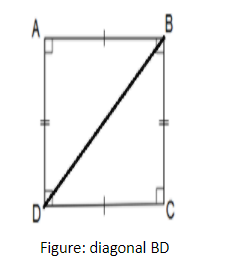
We know,
Apply Pythagoras theorem on
i.e. Pythagoras theorem: square of the hypotenuse is equal to the sum of the square of base and square of perpendicular.
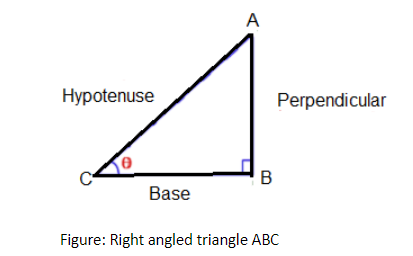
On substituting the corresponding values,
On simplification of the above values,
On further simplification, we get
Note:
Area of rectangle = length
The diagonal of the rectangle bisects its area.
Students may get confused in labeling hypotenuse, perpendicular, and base sides of the right-angled triangle. Remember that the hypotenuse is the longest side in the right-angled triangle. It is the side opposite to the right angle (or
Pythagoras theorem only applies to the right-angled triangle, not to every triangle.
We know that the opposite sides of the rectangle are equal. The angle between two adjacent sides is
The angle between two adjacent sides of a rectangle is
Remember that: Pythagoras theorem can be applied to every right-angled triangle.
Pythagoras theorem: square of the hypotenuse is equal to the sum of the square of base and square of perpendicular.
In a right-angled triangle, base and perpendicular are at the angle of
The comparison of two quantities in terms of ‘how many times’ is known as a ratio.
For example: There are 24 girls and 16 boys in a class. Ratio of the numbers of girls to the numbers of boys
Therefore, we can get equivalent ratios by multiplying or dividing the numerator and denominator by the same number.
A ratio can be written as a fraction, thus the ratio
Complete step by step solution:
Step 1: Draw the rectangle ABCD

Properties of rectangle:
LENGTH: Side
BREADTH: Side
Side
All the interior angles of the rectangle ABCD
Step 2: Given that
Perimeter of rectangle
Ratio of two unequal sides of rectangle
Step 3: multiply numerator and denominator by
Step 4: find length and breadth of rectangle using parameter of rectangle
Given Parameter of rectangle = 24 cm
On substituting the corresponding values,
On simplifying expressions,
On further simplification, we get
Hence, length
And breadth
Step 5: Calculate diagonal BD using Pythagoras theorem
Join the Vertex BD, i.e. diagonal of rectangle ABCD.

We know,
Apply Pythagoras theorem on
i.e. Pythagoras theorem: square of the hypotenuse is equal to the sum of the square of base and square of perpendicular.

On substituting the corresponding values,
On simplification of the above values,
On further simplification, we get
Note:
Area of rectangle = length
The diagonal of the rectangle bisects its area.
Students may get confused in labeling hypotenuse, perpendicular, and base sides of the right-angled triangle. Remember that the hypotenuse is the longest side in the right-angled triangle. It is the side opposite to the right angle (or
Pythagoras theorem only applies to the right-angled triangle, not to every triangle.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




